Repairable Systems Analysis Reference Example: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
No edit summary |
||
| Line 56: | Line 56: | ||
{{Reference_Example_Heading4|RGA}} | {{Reference_Example_Heading4|RGA}} | ||
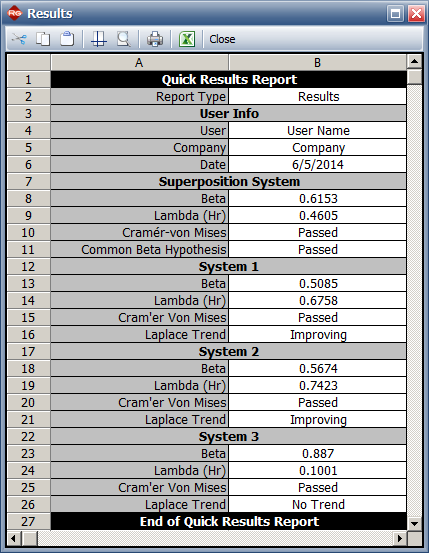
Since <math>\,\!S_{1}=S_{2}=S_{3}=0</math> and <math>\,\!T_{1}=T_{2}=T_{3}=200</math> then the maximum likelihood estimates of <math>\,\!\ | Since <math>\,\!S_{1}=S_{2}=S_{3}=0</math> and <math>\,\!T_{1}=T_{2}=T_{3}=200</math> then the maximum likelihood estimates of <math>\,\!\hat{\beta}</math> and <math>\,\!\hat{\lambda }</math> are given by: | ||
::<math>\begin{align} | ::<math>\begin{align} | ||
\ | \hat{\beta }=&\frac{\sum_{q=1}^{K}N_{q}}{\sum_{q=1}^{K}\sum_{i=1}^{N_{q}}ln \left(\frac{T}{X_{iq}}\right)}\\ | ||
\\ | \\ | ||
=&0.6153 | =&0.6153 | ||
| Line 67: | Line 67: | ||
::<math>\begin{align} | ::<math>\begin{align} | ||
\ | \hat{\lambda }=&\frac{{\underset{i=1}{\overset{N}{\mathop \sum }}N_{q}}}{KT^{\hat{\beta }}}\\ | ||
\\ | \\ | ||
=&0.4605 | =&0.4605 | ||
Revision as of 15:04, 13 June 2014
RGA_Reference_Examples_Banner.png