|
|
| Line 52: |
Line 52: |
|
| |
|
| {{Reference_Example_Heading3}} | | {{Reference_Example_Heading3}} |
| | |
| | The book has the following results: |
|
| |
|
| Beta = 0.615, Lambda = 0.461 | | Beta = 0.615, Lambda = 0.461 |
Revision as of 15:46, 13 June 2014
RGA_Reference_Examples_Banner.png
This example compares the results for a repairable systems analysis.
Reference Case
Crow, L.H., Reliability Analysis for Complex Repairable Systems, Reliability and Biometry: Statistical Analysis of Lifelength, pg. 385, 1974.
Data
The following table shows the data.
| System 1
|
System 2
|
System 3
|
| 4.3 |
0.1 |
8.4
|
| 4.4 |
5.6 |
32.4
|
| 10.2 |
18.6 |
44.7
|
| 23.5 |
19.5 |
48.4
|
| 23.8 |
24.2 |
50.6
|
| 26.4 |
26.7 |
73.6
|
| 74 |
45.1 |
98.7
|
| 77.1 |
45.8 |
112.2
|
| 92.1 |
72.7 |
129.8
|
| 197.2 |
75.7 |
136
|
|
98.6 |
195.8
|
|
120.1 |
|
|
161.8 |
|
|
180.6 |
|
|
190.8 |
|
Simulated Data for 3 Systems with End Time = 200 hours
Result
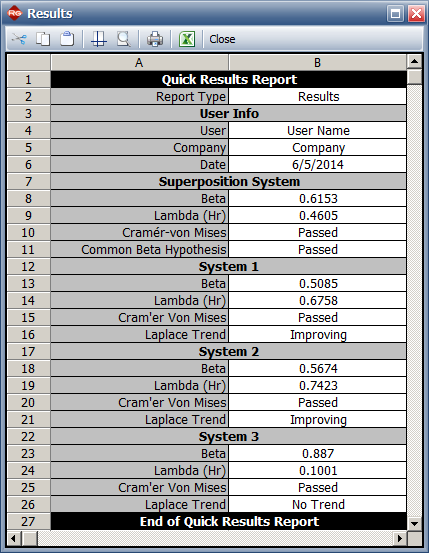
The book has the following results:
Beta = 0.615, Lambda = 0.461
Results in RGA
Since [math]\displaystyle{ \,\!S_{1}=S_{2}=S_{3}=0 }[/math] and [math]\displaystyle{ \,\!T_{1}=T_{2}=T_{3}=200 }[/math] then the maximum likelihood estimates of [math]\displaystyle{ \,\!\hat{\beta} }[/math] and [math]\displaystyle{ \,\!\hat{\lambda } }[/math] are given by:
- [math]\displaystyle{ \begin{align}
\hat{\beta }=&\frac{\sum_{q=1}^{K}N_{q}}{\sum_{q=1}^{K}\sum_{i=1}^{N_{q}}ln \left(\frac{T}{X_{iq}}\right)}\\
\\
=&0.6153
\end{align}\,\! }[/math]
- [math]\displaystyle{ \begin{align}
\hat{\lambda }=&\frac{{\underset{i=1}{\overset{N}{\mathop \sum }}N_{q}}}{KT^{\hat{\beta }}}\\
\\
=&0.4605
\end{align}\,\! }[/math]