|
|
| Line 17: |
Line 17: |
| !Voltage (kV)|style="width:50%" | | !Voltage (kV)|style="width:50%" |
| |- | | |- |
| | 15||219 | | | 15||219|style="width:50%" |
| |- | | |- |
| | 16||219 | | | 16||219 |
Revision as of 18:49, 11 June 2014
ALTA_Reference_Examples_Banner.png
This example compares the results for the IPL life stress relationship with a lognormal distribution.
Reference Case
The data set is from Example 19.10 on page 504 in book Statistical Methods for Reliability Data by Dr. Meeker and Dr. Escobar, John Wiley & Sons, 1998.
Data
A Mylar-Polyurethane Insulating structure was tested under several different voltage settings. The test data is given in the table shown next.
| Time Failed (Hr)
|
style="width:50%"
|
| 15 |
style="width:50%"
|
| 16 |
219
|
| 36 |
219
|
| 50 |
219
|
| 55 |
219
|
| 95 |
219
|
| 122 |
219
|
| 129 |
219
|
| 625 |
219
|
| 700 |
219
|
| 49 |
157.1
|
| 99 |
157.1
|
| 154.5 |
157.1
|
| 180 |
157.1
|
| 291 |
157.1
|
| 447 |
157.1
|
| 510 |
157.1
|
| 600 |
157.1
|
| 1656 |
157.1
|
| 1721 |
157.1
|
| 188 |
122.4
|
| 297 |
122.4
|
| 405 |
122.4
|
| 744 |
122.4
|
| 1218 |
122.4
|
| 1340 |
122.4
|
| 1715 |
122.4
|
| 3382 |
122.4
|
| 606 |
100.3
|
| 1012 |
100.3
|
| 2520 |
100.3
|
| 2610 |
100.3
|
| 3988 |
100.3
|
| 4100 |
100.3
|
| 5025 |
100.3
|
| 6842 |
100.3
|
Result
The following function is used for the Ln-Mean [math]\displaystyle{ \,\!\mu {}' }[/math]:
- [math]\displaystyle{ \,\!\mu {}'=\beta _{0}+\beta _{1}\times log\left ( V \right ) }[/math]
where V is the voltage and its natural log transform is used in the above life stress relation.
This function can be written in the following way:
- [math]\displaystyle{ \,\!e^{\mu {}'}=e^{\alpha _{0}+\alpha _{1}log\left ( V \right )} }[/math]
The above equation is the general log-linear model in ALTA. In ALTA, the coefficients are denoted by [math]\displaystyle{ \,\!\alpha _{i} }[/math].
In fact, the above model also can be expressed using the traditional IPL (inverse power law) model:
- [math]\displaystyle{ \,\!e^{\mu {}'}=\frac{1}{K\cdot V^{n}} }[/math]
where [math]\displaystyle{ \,\!K=e^{-\alpha _{0}} }[/math] and [math]\displaystyle{ \,\!n=-\alpha _{1} }[/math].
In the book, the following results are provided:
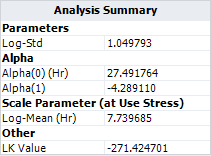
- ML estimations for the model parameters are: [math]\displaystyle{ \,\!\sigma =1.05 }[/math] , [math]\displaystyle{ \,\!\beta _{0}=27.5 }[/math] and [math]\displaystyle{ \,\!\beta _{1}=-4.29 }[/math].
- The standard deviation of each parameter are: [math]\displaystyle{ \,\!std\left ( \sigma \right )=0.12 }[/math] , [math]\displaystyle{ \,\!std\left ( \beta _{0} \right )=3.0 }[/math] and [math]\displaystyle{ \,\!std\left ( \beta _{1} \right )=0.6 }[/math].
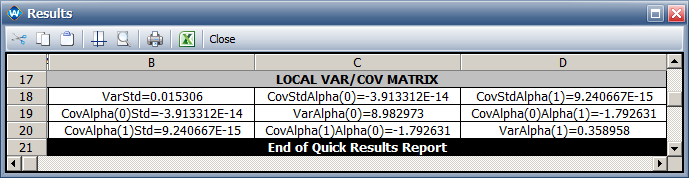
- Therefore, their variances are: [math]\displaystyle{ \,\!Var\left ( \sigma \right )=0.0144 }[/math] , [math]\displaystyle{ \,\!Var\left ( \beta _{0} \right )=9 }[/math] and [math]\displaystyle{ \,\!Var\left ( \beta _{1} \right )=0.36 }[/math].
- The log-likelihood value is -271.4.
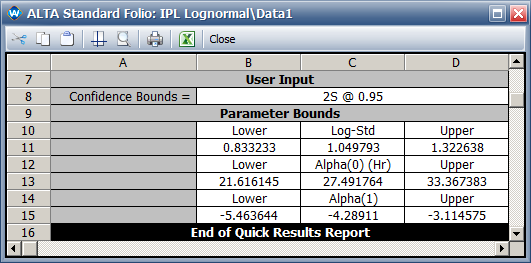
- The 95% two-sided confidence intervals are: for [math]\displaystyle{ \,\!\sigma }[/math] , it is [0.83, 1.32]; for [math]\displaystyle{ \,\!\beta _{0} }[/math] , it is [21.6, 33.4]; and for [math]\displaystyle{ \,\!\beta _{1} }[/math] , it is [-5.46, -3.11].
Results in ALTA
- ML estimations for the model parameters are:
- The variance and covariance matrix for model parameters is:
- The log-likelihood value is -271.4247.
- The 95% two-sided confidence intervals are: