Cumulative Damage Model for Progress Stress Profiles

From ReliaWiki
Jump to navigation Jump to search
ALTA Reference Examples Banner.png


New format available! This reference is now available in a new format that offers faster page load, improved display for calculations and images and more targeted search.

As of January 2024, this Reliawiki page will not continue to be updated. Please update all links and bookmarks to the latest references at ALTA examples and ALTA reference examples.



Cumulative Damage Model for Progress Stress Profiles

This example validates the results of a cumulative damage model for a ramp stress test in an ALTA standard folio.


Reference Case

The data set is from the table on page 232 in the book Accelerated Testing: Statistical Models, Test Plans, and Data Analysis by Dr. Nelson, John Wiley & Sons, 1990. The model of Eqn. (3.10) is used and the results are given in Section 3 of Table 3.2 on page 511.


Data

An accelerated test employed a pair of parallel disk electrodes immersed in insulating oil. The voltage V across the pair was increased linearly with time t at a specified rate, and then the voltage at oil breakdown was recorded. Since V = Rt (R is the ramp rate), the time to breakdown can be recorded. The breakdown time is also affected by the two electrode areas A. Three voltage linear rates and two electrode areas are used. The six stress profiles are:

Voltage Ramp Rate (Volts/sec) Area (Sq. Inch)
10 1
100 1
1000 1
10 9
100 9
1000 9


The following table shows the failure data for an area of 1 square inch.

Time to Failure Voltage Ramp Rate Time to Failure Voltage Ramp Rate Time to Failure Voltage Ramp Rate
41 10 46 100 55 1000
43 10 50 100 57 1000
42 10 39 100 59 1000
43 10 36 100 57 1000
44 10 47 100 55 1000
40 10 55 100 60 1000
38 10 49 100 53 1000
47 10 58 100 51 1000
43 10 50 100 57 1000
45 10 48 100 54 1000
38 10 53 100 57 1000
44 10 54 100 64 1000
49 10 55 100 53 1000
42 10 37 100 63 1000
42 10 53 100 51 1000
51 10 52 100 62 1000
39 10 53 100 62 1000
34 10 50 100 56 1000
41 10 52 100 62 1000
41 10 50 100 57 1000
35 10 45 100 41 1000
44 10 48 100 41 1000
46 10 53 100 51 1000
39 10 50 100 58 1000
41 10 43 100 59 1000
40 10 50 100 60 1000
52 10 42 100 58 1000
40 10 45 100 55 1000
35 10 47 100 59 1000
40 10 34 100 63 1000
39 10 46 100 63 1000
46 10 42 100 53 1000
47 10 46 100 63 1000
44 10 46 100 61 1000
41 10 52 100 59 1000
46 10 47 100 53 1000
46 10 53 100 60 1000
42 10 52 100 58 1000
45 10 45 100 62 1000
42 10 47 100 56 1000
44 10 43 100 69 1000
41 10 45 100 65 1000
44 10 54 100 51 1000
38 10 51 100 56 1000
36 10 46 100 55 1000
44 10 55 100 57 1000
50 10 44 100 54 1000
47 10 49 100 63 1000
49 10 49 100 65 1000
46 10 53 100 65 1000
34 10 53 100 56 1000
47 10 54 100 54 1000
49 10 53 100 65 1000
43 10 53 100 60 1000
43 10 51 100 60 1000
48 10 48 100 64 1000
34 10 49 100 60 1000
38 10 52 100 54 1000
47 10 45 100 57 1000
35 10 49 100 61 1000


The following table shows the failure data for an area of 9 square inch.

Time to Failure Voltage Ramp Rate Time to Failure Voltage Ramp Rate Time to Failure Voltage Ramp Rate
33 10 43 100 50 1000
37 10 42 100 53 1000
38 10 45 100 50 1000
38 10 48 100 49 1000
38 10 38 100 53 1000
37 10 44 100 51 1000
27 10 37 100 47 1000
42 10 44 100 44 1000
39 10 43 100 53 1000
38 10 42 100 42 1000
42 10 43 100 49 1000
32 10 49 100 46 1000
42 10 44 100 50 1000
40 10 45 100 38 1000
32 10 50 100 48 1000
38 10 44 100 43 1000
36 10 44 100 52 1000
42 10 45 100 53 1000
20 10 41 100 52 1000
37 10 48 100 48 1000
43 10 45 100 45 1000
40 10 48 100 53 1000
38 10 43 100 52 1000
43 10 49 100 50 1000
39 10 50 100 55 1000
41 10 45 100 50 1000
35 10 45 100 43 1000
41 10 46 100 52 1000
40 10 47 100 50 1000
32 10 42 100 54 1000
38 10 47 100 51 1000
40 10 48 100 40 1000
37 10 47 100 52 1000
29 10 48 100 53 1000
31 10 39 100 47 1000
41 10 49 100 45 1000
38 10 44 100 53 1000
36 10 47 100 47 1000
35 10 34 100 54 1000
40 10 41 100 50 1000
37 10 45 100 32 1000
41 10 48 100 48 1000
36 10 44 100 53 1000
39 10 47 100 52 1000
43 10 45 100 45 1000
42 10 50 100 48 1000
43 10 40 100 48 1000
43 10 47 100 51 1000
41 10 47 100 53 1000
44 10 43 100 48 1000
37 10 49 100 54 1000
43 10 45 100 51 1000
38 10 45 100 50 1000
40 10 45 100 54 1000
40 10 47 100 35 1000
38 10 39 100 56 1000
33 10 44 100 51 1000
40 10 37 100 48 1000
35 10 47 100 48 1000
41 10 48 100 46 1000

Result

The following life stress relationship and Weibull distribution are used to analyze the data. At a constant stress V, the [math]\displaystyle{ \eta\,\! }[/math] is:

[math]\displaystyle{ ln\left(\eta(V, A)\right) = \alpha_{0}+\alpha_{1}ln(V)+\alpha_{2}ln(A)\,\! }[/math]


where V is the voltage and A is the area.

The reliability function at time t and stress V is:

[math]\displaystyle{ R(t,V,A) = e^{-\left(\frac{t}{\eta(V,A)} \right)^\beta}\,\! }[/math]


When stress is varying with time, the reliability at time t is given as follows:

[math]\displaystyle{ R(t,V,A) = e^{-\left(\int_{0}^{t}\frac{1}{\eta(x,A)}dx\right)^\beta} }[/math]


In the book, the following results are provided:

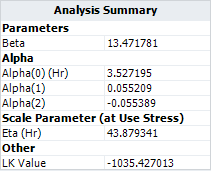
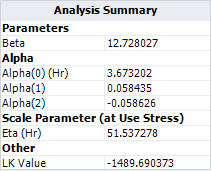
  • The ML solution for the parameters are given in Section 3 of Table 3.2 on page 511: [math]\displaystyle{ 1/\beta\,\! }[/math] = 0.07856677 ([math]\displaystyle{ \beta\,\! }[/math]=12.728027) ,  [math]\displaystyle{ \alpha_{0}\,\! }[/math] = 3.673202 ,   [math]\displaystyle{ \alpha_{1} = \,\! }[/math]0.05843506 ,  and  [math]\displaystyle{ \alpha_{2}=\,\! }[/math]-0.058626.
  • The maximum log likelihood is -1035.4269.


Results in ALTA

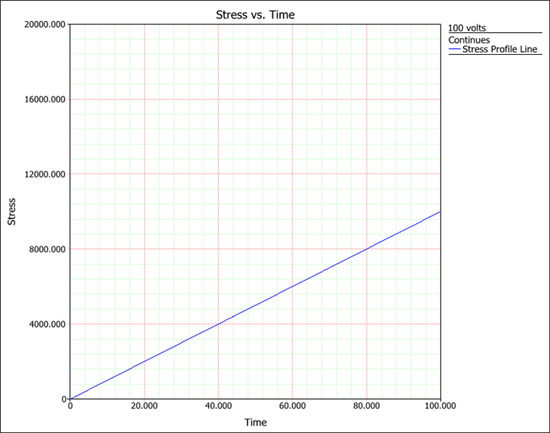
First, we create a stress profile in ALTA for each of three ramp rates: 10 volts/sec, 100 volts/sec and 1,000 volts/sec. For example, the following picture shows the stress profile for the 100 volts/sec ramp rate.

Cum Damage Stress Profile 100 volts.png


Note that the log transformation is used for voltage. At time 0, the voltage value will be 0 if the profile is defined as V(t) = 100*t; therefore, to define this stress profile, we add a small number (i.e., 0.001) to the voltage function.

The following picture shows the plot for this stress profile.

Cum Damage Stress Profile 100 volts plot.png


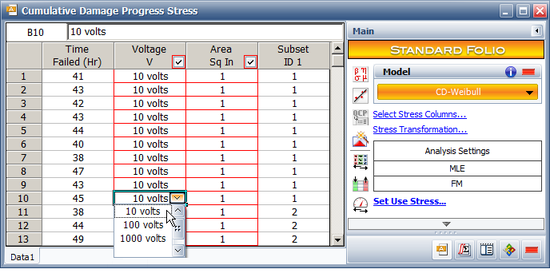
The next step is to enter the failure data into an ALTA standard folio and then use the stress profiles to define the stress values, as shown next.

Cum Damage Stress Profile folio.png


The log transformation is used for both stresses. The results in ALTA show that the calculated parameters are close to the results in the book, while the log likelihood value (LK Value) is exactly the same as the value given in the book, as shown next.

Cum Damage Stress Profile result1.png


Validate the Likelihood Value Calculation in ALTA

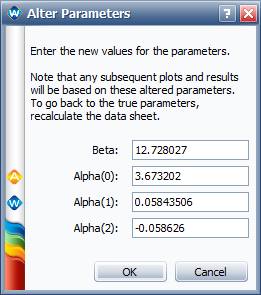
For the ML solution given in the book, the log likelihood value is -1035.4269. To validate what the LK value would be if the parameter values used in the book were calculated in ALTA, we use the Alter Parameters tool, as shown next.

Cum Damage Stress Profile alter parameters.png


The resulting LK Value is -1489.6904, as shown next.

Cum Damage Stress Profile alter parameters results.png


We need to validate the calculation for the log likelihood value in ALTA to make sure it is correct.

First, we define the life characteristic of the Weibull distribution as a function of the two stresses:

[math]\displaystyle{ C = \int^{t}_{0} \frac{1}{\eta(V,A)} dV = \int^{t}_{0} e^{-(\alpha_{0}+\alpha_{1} \cdot ln(R \cdot V)+\alpha_{2} \cdot ln A)} dV\,\! }[/math]


We get the equation for C as:

[math]\displaystyle{ C = e^{-\alpha_{0}} \cdot A^{-\alpha_{2}} \cdot R^{-\alpha_{1}} \cdot \frac{1}{1-\alpha_{1}} \cdot t^{1-\alpha_{1}}\,\! }[/math]


where R is the ramping rate for the voltage.

Therefore, the probability density function at failure time t is:

[math]\displaystyle{ f(t) = \beta \cdot e^{-C^{\beta}} \cdot C^{\beta} \cdot (1-\alpha_{1}) \cdot \frac{1}{t}\,\! }[/math]


The following table shows the value for the pdf and its log transformation for the first several failure times. The log likelihood value for all other failure times can be calculated using the same method. The total log likelihood value is the sum of all the individual log likelihood values.

Time to Failure Voltage Ramp Rate Area f(t) log-f(t)
41 10 1 0.069249 -2.67004
43 10 1 0.094154 -2.36283
42 10 1 0.08244 -2.49568
43 10 1 0.094154 -2.36283
44 10 1 0.102165 -2.28117
40 10 1 0.05623 -2.8783
38 10 1 0.034243 -3.37427
47 10 1 0.083568 -2.4821
43 10 1 0.094154 -2.36283
45 10 1 0.104043 -2.26295
… … … … …
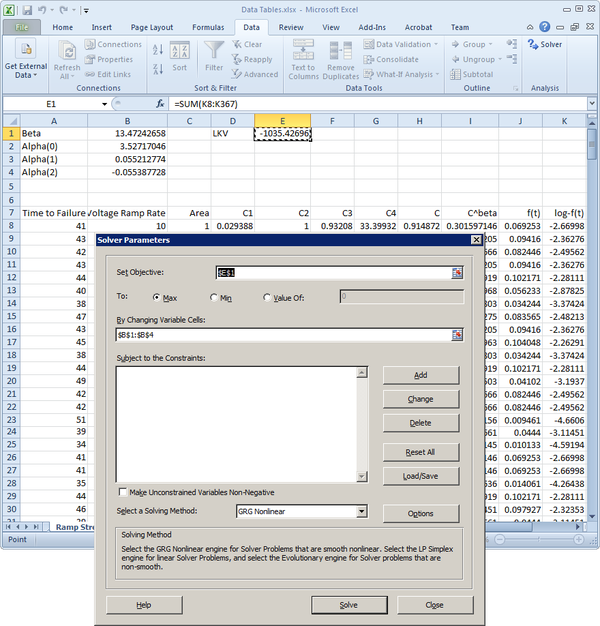
Total -1035.42696


The total likelihood value is LKV = -2.67004 -2.36283--2.49568…= -1035.42696.

We also can use the optimization tool in Excel to find the ML solutions for this data set. By using the GRG-nonlinear optimization method provided by the "Solver Add-in" program in Excel, the result in Excel is the same as the result in ALTA.

Cum Damage Stress Profile excel add in.png


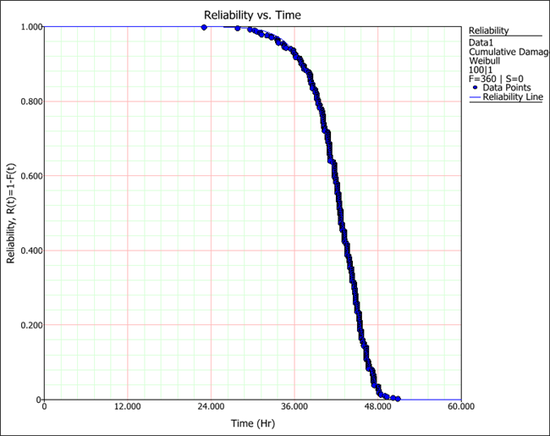
The following reliability plot shows that the predicted values (the line) from the model in ALTA can match the observed values (the points) very well.

Cum Damage Stress Profile plot.png
Retrieved from "https://www.reliawiki.com/index.php?title=Cumulative_Damage_Model_for_Progress_Stress_Profiles&oldid=60838"