Template:Example: Normal Distribution Likelihood Ratio Bound (Time)

Normal Distribution Likelihood Ratio Bound Example (Time)

For the data given in Example 5, determine the two-sided 80% confidence bounds on the time estimate for a reliability of 40%. The ML estimate for the time at [math]\displaystyle{ R(t)=40% }[/math] is 31.637.

Solution

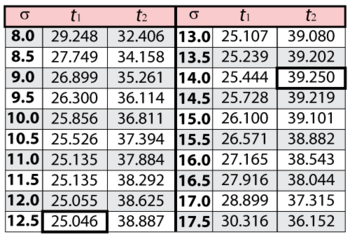
In this example, we are trying to determine the two-sided 80% confidence bounds on the time estimate of 31.637. This is accomplished by substituting [math]\displaystyle{ R=0.40 }[/math] and [math]\displaystyle{ \alpha =0.8 }[/math] into the likelihood ratio equation for the normal distribution, and varying [math]\displaystyle{ \sigma }[/math] until the maximum and minimum values of [math]\displaystyle{ t }[/math] are found. The following table gives the values of [math]\displaystyle{ t }[/math] based on given values of [math]\displaystyle{ \sigma }[/math] .

This data set is represented graphically in the following contour plot:

As can be determined from the table, the lowest calculated value for [math]\displaystyle{ t }[/math] is 25.046, while the highest is 39.250. These represent the 80% confidence limits on the time at which reliability is equal to 40%.