ALTA Test Plan Example
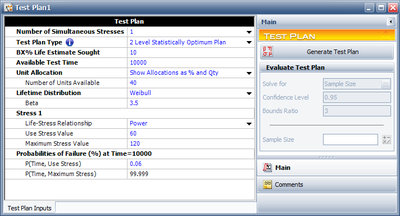
A reliability engineer is planning an accelerated test for a mechanical component. Torque is the only factor in the test. The purpose of the experiment is to estimate the [math]\displaystyle{ B10 }[/math] life (Time equivalent to Unreliability = 0.1) of the diodes. The reliability engineer wants to use a Two Level Statistically Optimum Plan because it would require fewer test chambers than a three level test plans. 40 units are available for the test. The mechanical component is assumed to follow a Weibull distribution with [math]\displaystyle{ \beta =3.5 }[/math] and a Power model is assumed for the life-stress relationship. The test is planned to last for 10,000 cycles. The engineer has estimated that there is a 0.06% probability that a unit will fail by 10,000 cycles at the use stress level of 60Nm. The highest level allowed in the test is 120Nm and a unit is estimated to fail with a probability of 99.999% at 120Nm. The following is the setup to generate the test plan in ALTA.
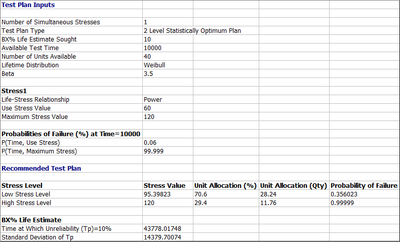
The Two Level Statistically Optimum Plan is shown next.
The Two Level Statistically Optimum Plan is to test 28.24 units at 95.39Nm and 11.76 units at 120Nm. The variance of the test at [math]\displaystyle{ B10 }[/math] is [math]\displaystyle{ Var({{T}_{p}}=B10)=StdDev{{({{T}_{p}}=B10)}^{2}}={{14380}^{2}}. }[/math]
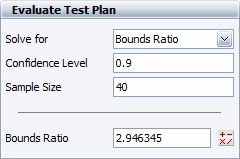
Now assume that a 90% confidence is desired and 40 units are to be used in the test, then the bounds ratio is calculated as 2.946345, as shown next.
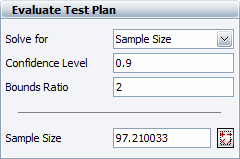
If this calculated bounds ratio is unsatisfactory, the analyst can calculate the required number of units that would meet a certain bounds ratio criterion. For example, if a bounds ratio of 2 is desired, the required sample size is calculated as 97.210033, as shown next.
If the sample size is kept at 40 units and a bounds ratio of 2 is desired, the equivalent confidence level we have in the test drops to 70.8629%, as shown next.