Example: Parametric RDA - Air Condition Unit

New format available! This reference is now available in a new format that offers faster page load, improved display for calculations and images and more targeted search.

As of January 2024, this Reliawiki page will not continue to be updated. Please update all links and bookmarks to the latest references at Weibull examples and Weibull reference examples.

---

The following table gives the failure times for the air conditioning unit of an aircraft. The observation ended by the time the last failure occurred. [3]

[math]\displaystyle{ \begin{matrix} \text{50} & \text{329} & \text{811} & \text{991} & \text{1489} \\ \text{94} & \text{332} & \text{899} & \text{1013} & \text{1512} \\ \text{196} & \text{347} & \text{945} & \text{1152} & \text{1525} \\ \text{268} & \text{544} & \text{950} & \text{1362} & \text{1539} \\ \text{290} & \text{732} & \text{955} & \text{1459} & {} \\ \end{matrix} }[/math]

1. Estimate the GRP model parameters using the Type I virtual age option.

2. Plot the failure number and instantaneous failure intensity vs. time with 90% two-sided confidence bounds.

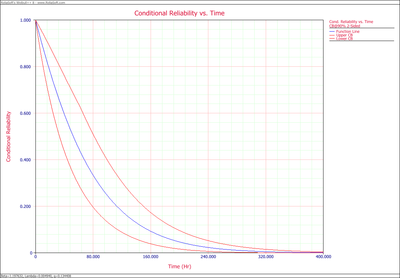
3. Plot the conditional reliability vs. time with 90% two-sided confidence bounds. The mission start time is 40 and mission time is varying.

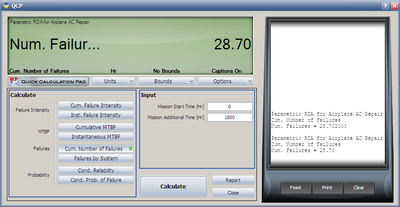
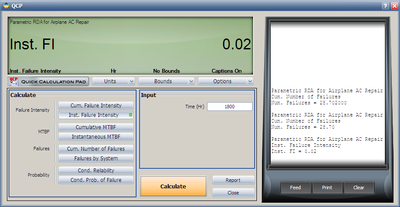
4. Using the QCP, calculate the expected failure number and expected instantaneous failure intensity by time 1800.

Solution

Enter the data into a parametric RDA folio in Weibull++. Choose 3 under Parameters and Type I under Settings. Keep the default simulation settings.

1. The estimated parameters are [math]\displaystyle{ \hat{\beta }=1.1976, }[/math] [math]\displaystyle{ \hat{\lambda }=4.94E-03, }[/math] [math]\displaystyle{ \hat{q}=0.1344 }[/math] .

2. The failure number and instantaneous failure intensity are given in the following plots.

3. The conditional reliability is plotted below.

4. Using QCP, the failure number and instantaneous failure intensity are: