Template:Stress-Strength Analysis in Design For Reliability

Stress-Strength Analysis in Design for Reliability

The stress distribution usually can be estimated from customer usage data. For example, the mileage per year of a passenger car or the load distribution for a beam. The strength distribution is affected by the material used in the component, the geometric dimensions and the manufacturing process. By adjusting these factors, a strength distribution that meets the reliability goal can be obtained. The reliability goal can be used to decide the distribution goal of the strength. As we know, the expected reliability is called from the following stress-strength calculation:

[math]\displaystyle{ R=P[Stress\le Strength]=\int_{0}^{\infty }{{{f}_{Stress}}(x)\cdot {{R}_{Strength}}(x)}dx }[/math]

Since the stress distribution can be estimated from customer usage data, [math]\displaystyle{ {f}_{Stress} }[/math] can be assumed to be known. For a given reliability goal, the strength distribution [math]\displaystyle{ {R}_{Strength} }[/math] is the only unknown and can be solved from the above equation. The following example explains how to use Weibull++ to determine the parameters for a strength distribution that are required to meet a specified reliability goal.

Example 2:

Assume that the stress distribution for a component is known to be a Weibull distribution with beta = 3 and eta = 2000. For the current design, the strength distribution is also a Weibull distribution with beta =1.5 and eta=4000. Evaluate the current reliability of the component. If the reliability does not meet the target reliability of 90%, determine what parameters would be required for the strength distribution in order to meet the specified target.

Solution

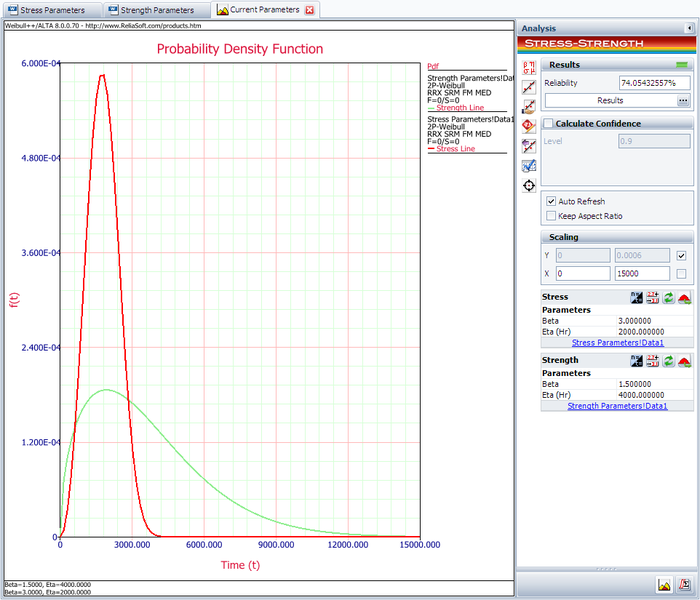
The following picture shows the stress-strength tool and the calculated reliability of the current design.

The result shows that the current reliability is about 74.0543%, which is below the target value of 90%. We need to use the Target Reliability Parameter Estimator to determine the parameters for the strength distribution that, when compared against the stress distribution, would result in the target reliability.

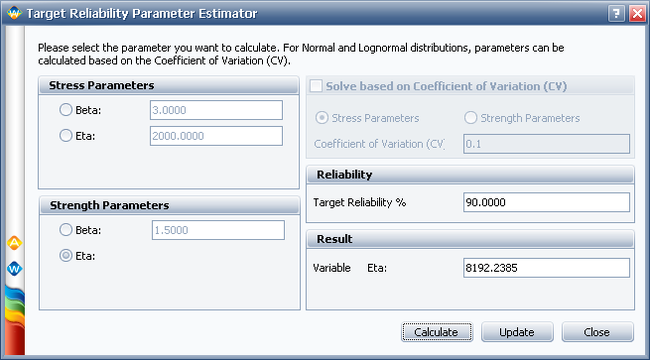
The following picture shows the Target Reliability Parameter Estimator window. In the Strength Parameters area, select eta. Set the Target Reliability to 90% and click Calculate. The calculated eta is 8192.2385 hours.

Click Update to perform the stress-strength analysis again using the altered parameters for the strength distribution. The following plot shows that the calculated reliability is 90%. Therefore, in order to meet the reliability requirement, the component must be redesigned such that the eta parameter of the strength distribution is at least 8192.2385 hours.