Template:Confidence bounds for rsa

Confidence Bounds for Repairable Systems Analysis

Bounds on [math]\displaystyle{ \beta }[/math]

Fisher Matrix Bounds

The parameter [math]\displaystyle{ \beta }[/math] must be positive, thus [math]\displaystyle{ \ln \beta }[/math] is approximately treated as being normally distributed.

[math]\displaystyle{ \frac{\ln (\widehat{\beta })-\ln (\beta )}{\sqrt{Var\left[ \ln (\widehat{\beta }) \right]}}\ \tilde{\ }\ N(0,1) }[/math]

[math]\displaystyle{ C{{B}_{\beta }}=\widehat{\beta }{{e}^{\pm {{z}_{\alpha }}\sqrt{Var(\widehat{\beta })}/\widehat{\beta }}} }[/math]

[math]\displaystyle{ \widehat{\beta }=\frac{\underset{q=1}{\overset{K}{\mathop{\sum }}}\,{{N}_{q}}}{\widehat{\lambda }\underset{q=1}{\overset{K}{\mathop{\sum }}}\,\left[ (T_{q}^{\widehat{\beta }}\ln ({{T}_{q}})-S_{q}^{\widehat{\beta }}\ln ({{S}_{q}}) \right]-\underset{q=1}{\overset{K}{\mathop{\sum }}}\,\underset{i=1}{\overset{{{N}_{q}}}{\mathop{\sum }}}\,\ln ({{X}_{i}}{{}_{q}})} }[/math]

All variance can be calculated using the Fisher Information Matrix.
[math]\displaystyle{ \Lambda }[/math] is the natural log-likelihood function.

[math]\displaystyle{ \Lambda =\underset{q=1}{\overset{K}{\mathop \sum }}\,\left[ {{N}_{q}}(\ln (\lambda )+\ln (\beta ))-\lambda (T_{q}^{\beta }-S_{q}^{\beta })+(\beta -1)\underset{i=1}{\overset{{{N}_{q}}}{\mathop \sum }}\,\ln ({{x}_{iq}}) \right] }[/math]

[math]\displaystyle{ \frac{{{\partial }^{2}}\Lambda }{\partial {{\lambda }^{2}}}=-\frac{\underset{q=1}{\overset{K}{\mathop{\sum }}}\,{{N}_{q}}}{{{\lambda }^{2}}} }[/math]

[math]\displaystyle{ \frac{{{\partial }^{2}}\Lambda }{\partial \lambda \partial \beta }=-\underset{q=1}{\overset{K}{\mathop \sum }}\,\left[ T_{q}^{\beta }\ln ({{T}_{q}})-S_{q}^{\beta }\ln ({{S}_{q}}) \right] }[/math]

[math]\displaystyle{ \frac{{{\partial }^{2}}\Lambda }{\partial {{\beta }^{2}}}=-\frac{\underset{q=1}{\overset{K}{\mathop{\sum }}}\,{{N}_{q}}}{{{\beta }^{2}}}-\lambda \underset{q=1}{\overset{K}{\mathop \sum }}\,\left[ T_{q}^{\beta }{{(\ln ({{T}_{q}}))}^{2}}-S_{q}^{\beta }{{(\ln ({{S}_{q}}))}^{2}} \right] }[/math]

Crow Bounds

Calculate the conditional maximum likelihood estimate of [math]\displaystyle{ \tilde{\beta } }[/math] :

[math]\displaystyle{ \tilde{\beta }=\frac{\underset{q=1}{\overset{K}{\mathop{\sum }}}\,{{M}_{q}}}{\underset{q=1}{\overset{K}{\mathop{\sum }}}\,\underset{i=1}{\overset{M}{\mathop{\sum }}}\,\ln \left( \tfrac{{{T}_{q}}}{{{X}_{iq}}} \right)} }[/math]

The Crow 2-sided [math]\displaystyle{ (1-a) }[/math] 100-percent confidence bounds on [math]\displaystyle{ \beta }[/math] are:

[math]\displaystyle{ \begin{align} & {{\beta }_{L}}= & \tilde{\beta }\frac{\chi _{\tfrac{\alpha }{2},2M}^{2}}{2M} \\ & {{\beta }_{U}}= & \tilde{\beta }\frac{\chi _{1-\tfrac{\alpha }{2},2M}^{2}}{2M} \end{align} }[/math]

Bounds on [math]\displaystyle{ \lambda }[/math]

Fisher Matrix Bounds

The parameter [math]\displaystyle{ \lambda }[/math] must be positive, thus [math]\displaystyle{ \ln \lambda }[/math] is approximately treated as being normally distributed. These bounds are based on:

[math]\displaystyle{ \frac{\ln (\widehat{\lambda })-\ln (\lambda )}{\sqrt{Var\left[ \ln (\widehat{\lambda }) \right]}}\ \tilde{\ }\ N(0,1) }[/math]

The approximate confidence bounds on [math]\displaystyle{ \lambda }[/math] are given as:

[math]\displaystyle{ C{{B}_{\lambda }}=\widehat{\lambda }{{e}^{\pm {{z}_{\alpha }}\sqrt{Var(\widehat{\lambda })}/\widehat{\lambda }}} }[/math]

where [math]\displaystyle{ \widehat{\lambda }=\tfrac{n}{T_{K}^{{\hat{\beta }}}} }[/math] . The variance calculation is the same as Eqns. (var1), (var2) and (var3).

Crow Bounds

Time Terminated
The confidence bounds on [math]\displaystyle{ \lambda }[/math] for time terminated data are calculated using:

[math]\displaystyle{ \begin{align} & {{\lambda }_{L}}= & \frac{\chi _{\tfrac{\alpha }{2},2N}^{2}}{2\cdot \underset{q=1}{\overset{K}{\mathop{\sum }}}\,T_{q}^{^{\beta }}} \\ & {{\lambda }_{u}}= & \frac{\chi _{1-\tfrac{\alpha }{2},2N+2}^{2}}{2\cdot \underset{q=1}{\overset{K}{\mathop{\sum }}}\,T_{q}^{^{\beta }}} \end{align} }[/math]

Failure Terminated
The confidence bounds on [math]\displaystyle{ \lambda }[/math] for failure terminated data are calculated using:

[math]\displaystyle{ \begin{align} & {{\lambda }_{L}}= & \frac{\chi _{\tfrac{\alpha }{2},2N}^{2}}{2\cdot \underset{q=1}{\overset{K}{\mathop{\sum }}}\,T_{q}^{^{\beta }}} \\ & {{\lambda }_{u}}= & \frac{\chi _{1-\tfrac{\alpha }{2},2N}^{2}}{2\cdot \underset{q=1}{\overset{K}{\mathop{\sum }}}\,T_{q}^{^{\beta }}} \end{align} }[/math]

Bounds on Growth Rate

Since the growth rate is equal to [math]\displaystyle{ 1-\beta }[/math] , the confidence bounds are:

[math]\displaystyle{ \begin{align} & Gr.\text{ }Rat{{e}_{L}}= & 1-{{\beta }_{U}} \\ & Gr.\text{ }Rat{{e}_{U}}= & 1-{{\beta }_{L}} \end{align} }[/math]

If Fisher Matrix confidence bounds are used then [math]\displaystyle{ {{\beta }_{L}} }[/math] and [math]\displaystyle{ {{\beta }_{U}} }[/math] are obtained from Eqn. (betafc). If Crow bounds are used then [math]\displaystyle{ {{\beta }_{L}} }[/math] and [math]\displaystyle{ {{\beta }_{U}} }[/math] are obtained from Eqn. (betacc).

Bounds on Cumulative MTBF

Fisher Matrix Bounds

The cumulative MTBF, [math]\displaystyle{ {{m}_{c}}(t) }[/math] , must be positive, thus [math]\displaystyle{ \ln {{m}_{c}}(t) }[/math] is approximately treated as being normally distributed.

[math]\displaystyle{ \frac{\ln ({{\widehat{m}}_{c}}(t))-\ln ({{m}_{c}}(t))}{\sqrt{Var\left[ \ln ({{\widehat{m}}_{c}}(t)) \right]}}\ \tilde{\ }\ N(0,1) }[/math]

The approximate confidence bounds on the cumulative MTBF are then estimated from:

[math]\displaystyle{ CB={{\widehat{m}}_{c}}(t){{e}^{\pm {{z}_{\alpha }}\sqrt{Var({{\widehat{m}}_{c}}(t))}/{{\widehat{m}}_{c}}(t)}} }[/math]

where:

[math]\displaystyle{ {{\widehat{m}}_{c}}(t)=\frac{1}{\widehat{\lambda }}{{t}^{1-\widehat{\beta }}} }[/math]

[math]\displaystyle{ \begin{align} & Var({{\widehat{m}}_{c}}(t))= & {{\left( \frac{\partial {{m}_{c}}(t)}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial {{m}_{c}}(t)}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda }) \\ & & +2\left( \frac{\partial {{m}_{c}}(t)}{\partial \beta } \right)\left( \frac{\partial {{m}_{c}}(t)}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda })\, \end{align} }[/math]

The variance calculation is the same as Eqns. (var1), (var2) and (var3).

[math]\displaystyle{ \begin{align} & \frac{\partial {{m}_{c}}(t)}{\partial \beta }= & -\frac{1}{\widehat{\lambda }}{{t}^{1-\widehat{\beta }}}\ln (t) \\ & \frac{\partial {{m}_{c}}(t)}{\partial \lambda }= & -\frac{1}{{{\widehat{\lambda }}^{2}}}{{t}^{1-\widehat{\beta }}} \end{align} }[/math]

Crow Bounds

To calculate the Crow confidence bounds on cumulative MTBF, first calculate the Crow cumulative failure intensity confidence bounds:

[math]\displaystyle{ C{{(t)}_{L}}=\frac{\chi _{\tfrac{\alpha }{2},2N}^{2}}{2\cdot t} }[/math]

[math]\displaystyle{ C{{(t)}_{u}}=\frac{\chi _{1-\tfrac{\alpha }{2},2N+2}^{2}}{2\cdot t} }[/math]

Then

[math]\displaystyle{ \begin{align} & {{[MTB{{F}_{c}}]}_{L}}= & \frac{1}{C{{(t)}_{U}}} \\ & {{[MTB{{F}_{c}}]}_{U}}= & \frac{1}{C{{(t)}_{L}}} \end{align} }[/math]

Bounds on Instantaneous MTBF

Fisher Matrix Bounds

The instantaneous MTBF, [math]\displaystyle{ {{m}_{i}}(t) }[/math] , must be positive, thus [math]\displaystyle{ \ln {{m}_{i}}(t) }[/math] is approximately treated as being normally distributed.

[math]\displaystyle{ \frac{\ln ({{\widehat{m}}_{i}}(t))-\ln ({{m}_{i}}(t))}{\sqrt{Var\left[ \ln ({{\widehat{m}}_{i}}(t)) \right]}}\ \tilde{\ }\ N(0,1) }[/math]

The approximate confidence bounds on the instantaneous MTBF are then estimated from:

[math]\displaystyle{ CB={{\widehat{m}}_{i}}(t){{e}^{\pm {{z}_{\alpha }}\sqrt{Var({{\widehat{m}}_{i}}(t))}/{{\widehat{m}}_{i}}(t)}} }[/math]

where:

[math]\displaystyle{ {{\widehat{m}}_{i}}(t)=\frac{1}{\lambda \beta {{t}^{\beta -1}}} }[/math]

[math]\displaystyle{ \begin{align} & Var({{\widehat{m}}_{i}}(t))= & {{\left( \frac{\partial {{m}_{i}}(t)}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial {{m}_{i}}(t)}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda }) \\ & & +2\left( \frac{\partial {{m}_{i}}(t)}{\partial \beta } \right)\left( \frac{\partial {{m}_{i}}(t)}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda }) \end{align} }[/math]

The variance calculation is the same as (var1), (var2) and (var3).

[math]\displaystyle{ \begin{align} & \frac{\partial {{m}_{i}}(t)}{\partial \beta }= & -\frac{1}{\widehat{\lambda }{{\widehat{\beta }}^{2}}}{{t}^{1-\widehat{\beta }}}-\frac{1}{\widehat{\lambda }\widehat{\beta }}{{t}^{1-\widehat{\beta }}}\ln (t) \\ & \frac{\partial {{m}_{i}}(t)}{\partial \lambda }= & -\frac{1}{{{\widehat{\lambda }}^{2}}\widehat{\beta }}{{t}^{1-\widehat{\beta }}} \end{align} }[/math]

Crow Bounds

Failure Terminated Data
To calculate the bounds for failure terminated data, consider the following equation:

[math]\displaystyle{ G(\mu |n)=\mathop{}_{0}^{\infty }\frac{{{e}^{-x}}{{x}^{n-2}}}{(n-2)!}\underset{i=0}{\overset{n-1}{\mathop \sum }}\,\frac{1}{i!}{{\left( \frac{\mu }{x} \right)}^{i}}\exp (-\frac{\mu }{x})\,dx }[/math]

Find the values [math]\displaystyle{ {{p}_{1}} }[/math] and [math]\displaystyle{ {{p}_{2}} }[/math] by finding the solution [math]\displaystyle{ c }[/math] to [math]\displaystyle{ G({{n}^{2}}/c|n)=\xi }[/math] for [math]\displaystyle{ \xi =\tfrac{\alpha }{2} }[/math] and [math]\displaystyle{ \xi =1-\tfrac{\alpha }{2} }[/math] , respectively. If using the biased parameters, [math]\displaystyle{ \hat{\beta } }[/math] and [math]\displaystyle{ \hat{\lambda } }[/math] , then the upper and lower confidence bounds are:

[math]\displaystyle{ \begin{align} & {{[MTB{{F}_{i}}]}_{L}}= & MTB{{F}_{i}}\cdot {{p}_{1}} \\ & {{[MTB{{F}_{i}}]}_{U}}= & MTB{{F}_{i}}\cdot {{p}_{2}} \end{align} }[/math]

where [math]\displaystyle{ MTB{{F}_{i}}=\tfrac{1}{\hat{\lambda }\hat{\beta }{{t}^{\hat{\beta }-1}}} }[/math] . If using the unbiased parameters, [math]\displaystyle{ \bar{\beta } }[/math] and [math]\displaystyle{ \bar{\lambda } }[/math] , then the upper and lower confidence bounds are:

[math]\displaystyle{ \begin{align} & {{[MTB{{F}_{i}}]}_{L}}= & MTB{{F}_{i}}\cdot \left( \frac{N-2}{N} \right)\cdot {{p}_{1}} \\ & {{[MTB{{F}_{i}}]}_{U}}= & MTB{{F}_{i}}\cdot \left( \frac{N-2}{N} \right)\cdot {{p}_{2}} \end{align} }[/math]

where [math]\displaystyle{ MTB{{F}_{i}}=\tfrac{1}{\hat{\lambda }\hat{\beta }{{t}^{\hat{\beta }-1}}} }[/math] .

Time Terminated Data
To calculate the bounds for time terminated data, consider the following equation where [math]\displaystyle{ {{I}_{1}}(.) }[/math] is the modified Bessel function of order one:

[math]\displaystyle{ H(x|k)=\underset{j=1}{\overset{k}{\mathop \sum }}\,\frac{{{x}^{2j-1}}}{{{2}^{2j-1}}(j-1)!j!{{I}_{1}}(x)} }[/math]

Find the values [math]\displaystyle{ {{\Pi }_{1}} }[/math] and [math]\displaystyle{ {{\Pi }_{2}} }[/math] by finding the solution [math]\displaystyle{ x }[/math] to [math]\displaystyle{ H(x|k)=\tfrac{\alpha }{2} }[/math] and [math]\displaystyle{ H(x|k)=1-\tfrac{\alpha }{2} }[/math] in the cases corresponding to the lower and upper bounds, respectively.
Calculate [math]\displaystyle{ \Pi =\tfrac{{{n}^{2}}}{4{{x}^{2}}} }[/math] for each case. If using the biased parameters, [math]\displaystyle{ \hat{\beta } }[/math] and [math]\displaystyle{ \hat{\lambda } }[/math] , then the upper and lower confidence bounds are:

[math]\displaystyle{ \begin{align} & {{[MTB{{F}_{i}}]}_{L}}= & MTB{{F}_{i}}\cdot {{\Pi }_{1}} \\ & {{[MTB{{F}_{i}}]}_{U}}= & MTB{{F}_{i}}\cdot {{\Pi }_{2}} \end{align} }[/math]

where [math]\displaystyle{ MTB{{F}_{i}}=\tfrac{1}{\hat{\lambda }\hat{\beta }{{t}^{\hat{\beta }-1}}} }[/math] . If using the unbiased parameters, [math]\displaystyle{ \bar{\beta } }[/math] and [math]\displaystyle{ \bar{\lambda } }[/math] , then the upper and lower confidence bounds are:

[math]\displaystyle{ \begin{align} & {{[MTB{{F}_{i}}]}_{L}}= & MTB{{F}_{i}}\cdot \left( \frac{N-1}{N} \right)\cdot {{\Pi }_{1}} \\ & {{[MTB{{F}_{i}}]}_{U}}= & MTB{{F}_{i}}\cdot \left( \frac{N-1}{N} \right)\cdot {{\Pi }_{2}} \end{align} }[/math]

where [math]\displaystyle{ MTB{{F}_{i}}=\tfrac{1}{\hat{\lambda }\hat{\beta }{{t}^{\hat{\beta }-1}}} }[/math] .

Bounds on Cumulative Failure Intensity

Fisher Matrix Bounds

The cumulative failure intensity, [math]\displaystyle{ {{\lambda }_{c}}(t) }[/math] must be positive, thus [math]\displaystyle{ \ln {{\lambda }_{c}}(t) }[/math] is approximately treated as being normally distributed.

[math]\displaystyle{ \frac{\ln ({{\widehat{\lambda }}_{c}}(t))-\ln ({{\lambda }_{c}}(t))}{\sqrt{Var\left[ \ln ({{\widehat{\lambda }}_{c}}(t)) \right]}}\ \tilde{\ }\ N(0,1) }[/math]

The approximate confidence bounds on the cumulative failure intensity are then estimated using:

[math]\displaystyle{ CB={{\widehat{\lambda }}_{c}}(t){{e}^{\pm {{z}_{\alpha }}\sqrt{Var({{\widehat{\lambda }}_{c}}(t))}/{{\widehat{\lambda }}_{c}}(t)}} }[/math]

where:

[math]\displaystyle{ {{\widehat{\lambda }}_{c}}(t)=\widehat{\lambda }{{t}^{\widehat{\beta }-1}} }[/math]

and:

[math]\displaystyle{ \begin{align} & Var({{\widehat{\lambda }}_{c}}(t))= & {{\left( \frac{\partial {{\lambda }_{c}}(t)}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial {{\lambda }_{c}}(t)}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda }) \\ & & +2\left( \frac{\partial {{\lambda }_{c}}(t)}{\partial \beta } \right)\left( \frac{\partial {{\lambda }_{c}}(t)}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda }) \end{align} }[/math]

The variance calculation is the same as Eqns. (var1), (var2) and (var3):

[math]\displaystyle{ \begin{align} & \frac{\partial {{\lambda }_{c}}(t)}{\partial \beta }= & \widehat{\lambda }{{t}^{\widehat{\beta }-1}}\ln (t) \\ & \frac{\partial {{\lambda }_{c}}(t)}{\partial \lambda }= & {{t}^{\widehat{\beta }-1}} \end{align} }[/math]

Crow Bounds

The Crow cumulative failure intensity confidence bounds are given by:

[math]\displaystyle{ C{{(t)}_{L}}=\frac{\chi _{\tfrac{\alpha }{2},2N}^{2}}{2\cdot t} }[/math]

[math]\displaystyle{ C{{(t)}_{u}}=\frac{\chi _{1-\tfrac{\alpha }{2},2N+2}^{2}}{2\cdot t} }[/math]

Bounds on Instantaneous Failure Intensity

Fisher Matrix Bounds

The instantaneous failure intensity, [math]\displaystyle{ {{\lambda }_{i}}(t) }[/math] , must be positive, thus [math]\displaystyle{ \ln {{\lambda }_{i}}(t) }[/math] is approximately treated as being normally distributed.

[math]\displaystyle{ \frac{\ln ({{\widehat{\lambda }}_{i}}(t))-\ln ({{\lambda }_{i}}(t))}{\sqrt{Var\left[ \ln ({{\widehat{\lambda }}_{i}}(t)) \right]}}\sim N(0,1) }[/math]

The approximate confidence bounds on the instantaneous failure intensity are then estimated from:

[math]\displaystyle{ CB={{\widehat{\lambda }}_{i}}(t){{e}^{\pm {{z}_{\alpha }}\sqrt{Var({{\widehat{\lambda }}_{i}}(t))}/{{\widehat{\lambda }}_{i}}(t)}} }[/math]

where [math]\displaystyle{ {{\lambda }_{i}}(t)=\lambda \beta {{t}^{\beta -1}} }[/math] and:

[math]\displaystyle{ \begin{align} & Var({{\widehat{\lambda }}_{i}}(t))= & {{\left( \frac{\partial {{\lambda }_{i}}(t)}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial {{\lambda }_{i}}(t)}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda }) \\ & & +2\left( \frac{\partial {{\lambda }_{i}}(t)}{\partial \beta } \right)\left( \frac{\partial {{\lambda }_{i}}(t)}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda }) \end{align} }[/math]

The variance calculation is the same as Eqns. (var1), (var2) and (var3):

[math]\displaystyle{ \begin{align} & \frac{\partial {{\lambda }_{i}}(t)}{\partial \beta }= & \hat{\lambda }{{t}^{\widehat{\beta }-1}}+\hat{\lambda }\hat{\beta }{{t}^{\widehat{\beta }-1}}\ln (t) \\ & \frac{\partial {{\lambda }_{i}}(t)}{\partial \lambda }= & \widehat{\beta }{{t}^{\widehat{\beta }-1}} \end{align} }[/math]

Crow Bounds

The Crow instantaneous failure intensity confidence bounds are given as:

[math]\displaystyle{ \begin{align} & {{[{{\lambda }_{i}}(t)]}_{L}}= & \frac{1}{{{[MTB{{F}_{i}}]}_{U}}} \\ & {{[{{\lambda }_{i}}(t)]}_{U}}= & \frac{1}{{{[MTB{{F}_{i}}]}_{L}}} \end{align} }[/math]

Bounds on Time Given Cumulative MTBF

Fisher Matrix Bounds

The time, [math]\displaystyle{ T }[/math] , must be positive, thus [math]\displaystyle{ \ln T }[/math] is approximately treated as being normally distributed.

[math]\displaystyle{ \frac{\ln (\widehat{T})-\ln (T)}{\sqrt{Var\left[ \ln (\widehat{T}) \right]}}\ \tilde{\ }\ N(0,1) }[/math]

The confidence bounds on the time are given by:

[math]\displaystyle{ CB=\widehat{T}{{e}^{\pm {{z}_{\alpha }}\sqrt{Var(\widehat{T})}/\widehat{T}}} }[/math]

where:

[math]\displaystyle{ Var(\widehat{T})={{\left( \frac{\partial T}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial T}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda })+2\left( \frac{\partial T}{\partial \beta } \right)\left( \frac{\partial T}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda }) }[/math]

The variance calculation is the same as Eqns. (var1), (var2) and (var3).

[math]\displaystyle{ \widehat{T}={{(\lambda \cdot {{m}_{c}})}^{1/(1-\beta )}} }[/math]

[math]\displaystyle{ \begin{align} & \frac{\partial T}{\partial \beta }= & \frac{{{(\lambda \cdot {{m}_{c}})}^{1/(1-\beta )}}\ln (\lambda \cdot {{m}_{c}})}{{{(1-\beta )}^{2}}} \\ & \frac{\partial T}{\partial \lambda }= & \frac{{{(\lambda \cdot {{m}_{c}})}^{1/(1-\beta )}}}{\lambda (1-\beta )} \end{align} }[/math]

Crow Bounds

Step 1: Calculate:

[math]\displaystyle{ \hat{T}={{\left( \frac{{{\lambda }_{c}}(T)}{{\hat{\lambda }}} \right)}^{\tfrac{1}{\beta -1}}} }[/math]

Step 2: Estimate the number of failures:

[math]\displaystyle{ N(\hat{T})=\hat{\lambda }{{\hat{T}}^{{\hat{\beta }}}} }[/math]

Step 3: Obtain the confidence bounds on time given the cumulative failure intensity by solving for [math]\displaystyle{ {{t}_{l}} }[/math] and [math]\displaystyle{ {{t}_{u}} }[/math] in the following equations:

[math]\displaystyle{ \begin{align} & {{t}_{l}}= & \frac{\chi _{\tfrac{\alpha }{2},2N}^{2}}{2\cdot {{\lambda }_{c}}(T)} \\ & {{t}_{u}}= & \frac{\chi _{1-\tfrac{\alpha }{2},2N+2}^{2}}{2\cdot {{\lambda }_{c}}(T)} \end{align} }[/math]

Bounds on Time Given Instantaneous MTBF

Fisher Matrix Bounds

The time, [math]\displaystyle{ T }[/math] , must be positive, thus [math]\displaystyle{ \ln T }[/math] is approximately treated as being normally distributed.

[math]\displaystyle{ \frac{\ln (\widehat{T})-\ln (T)}{\sqrt{Var\left[ \ln (\widehat{T}) \right]}}\ \tilde{\ }\ N(0,1) }[/math]

The confidence bounds on the time are given by:

[math]\displaystyle{ CB=\widehat{T}{{e}^{\pm {{z}_{\alpha }}\sqrt{Var(\widehat{T})}/\widehat{T}}} }[/math]

where:

[math]\displaystyle{ Var(\widehat{T})={{\left( \frac{\partial T}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial T}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda })+2\left( \frac{\partial T}{\partial \beta } \right)\left( \frac{\partial T}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda }) }[/math]

The variance calculation is the same as Eqns. (var1), (var2) and (var3).

[math]\displaystyle{ \widehat{T}={{(\lambda \beta \cdot MTB{{F}_{i}})}^{1/(1-\beta )}} }[/math]

[math]\displaystyle{ \begin{align} & \frac{\partial T}{\partial \beta }= & {{\left( \lambda \beta \cdot MTB{{F}_{i}} \right)}^{1/(1-\beta )}}[\frac{1}{{{(1-\beta )}^{2}}}\ln (\lambda \beta \cdot MTB{{F}_{i}})+\frac{1}{\beta (1-\beta )}] \\ & \frac{\partial T}{\partial \lambda }= & \frac{{{(\lambda \beta \cdot MTB{{F}_{i}})}^{1/(1-\beta )}}}{\lambda (1-\beta )} \end{align} }[/math]

Crow Bounds

Step 1: Calculate the confidence bounds on the instantaneous MTBF as presented in Section 5.5.2.
Step 2: Calculate the bounds on time as follows.

Failure Terminated Data

[math]\displaystyle{ \hat{T}={{(\frac{\lambda \beta \cdot MTB{{F}_{i}}}{c})}^{1/(1-\beta )}} }[/math]

So the lower an upper bounds on time are:

[math]\displaystyle{ {{\hat{T}}_{L}}={{(\frac{\lambda \beta \cdot MTB{{F}_{i}}}{{{c}_{1}}})}^{1/(1-\beta )}} }[/math]

[math]\displaystyle{ {{\hat{T}}_{U}}={{(\frac{\lambda \beta \cdot MTB{{F}_{i}}}{{{c}_{2}}})}^{1/(1-\beta )}} }[/math]

Time Terminated Data

[math]\displaystyle{ \hat{T}={{(\frac{\lambda \beta \cdot MTB{{F}_{i}}}{\Pi })}^{1/(1-\beta )}} }[/math]

So the lower and upper bounds on time are:

[math]\displaystyle{ {{\hat{T}}_{L}}={{(\frac{\lambda \beta \cdot MTB{{F}_{i}}}{{{\Pi }_{1}}})}^{1/(1-\beta )}} }[/math]

[math]\displaystyle{ {{\hat{T}}_{U}}={{(\frac{\lambda \beta \cdot MTB{{F}_{i}}}{{{\Pi }_{2}}})}^{1/(1-\beta )}} }[/math]

Bounds on Time Given Cumulative Failure Intensity

Fisher Matrix Bounds

The time, [math]\displaystyle{ T }[/math] , must be positive, thus [math]\displaystyle{ \ln T }[/math] is approximately treated as being normally distributed.

[math]\displaystyle{ \frac{\ln (\widehat{T})-\ln (T)}{\sqrt{Var\left[ \ln \widehat{T} \right]}}\ \tilde{\ }\ N(0,1) }[/math]

The confidence bounds on the time are given by:

[math]\displaystyle{ CB=\widehat{T}{{e}^{\pm {{z}_{\alpha }}\sqrt{Var(\widehat{T})}/\widehat{T}}} }[/math]

where:

[math]\displaystyle{ Var(\widehat{T})={{\left( \frac{\partial T}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial T}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda })+2\left( \frac{\partial T}{\partial \beta } \right)\left( \frac{\partial T}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda }) }[/math]

The variance calculation is the same as Eqns. (var1), (var2) and (var3):

[math]\displaystyle{ \widehat{T}={{\left( \frac{{{\lambda }_{c}}(T)}{\lambda } \right)}^{1/(\beta -1)}} }[/math]

[math]\displaystyle{ \begin{align} & \frac{\partial T}{\partial \beta }= & \frac{-{{\left( \tfrac{{{\lambda }_{c}}(T)}{\lambda } \right)}^{1/(\beta -1)}}\ln \left( \tfrac{{{\lambda }_{c}}(T)}{\lambda } \right)}{{{(1-\beta )}^{2}}} \\ & \frac{\partial T}{\partial \lambda }= & {{\left( \frac{{{\lambda }_{c}}(T)}{\lambda } \right)}^{1/(\beta -1)}}\frac{1}{\lambda (1-\beta )} \end{align} }[/math]

Crow Bounds

Step 1: Calculate:

[math]\displaystyle{ \hat{T}={{\left( \frac{{{\lambda }_{c}}(T)}{{\hat{\lambda }}} \right)}^{\tfrac{1}{\beta -1}}} }[/math]

Step 2: Estimate the number of failures:

[math]\displaystyle{ N(\hat{T})=\hat{\lambda }{{\hat{T}}^{{\hat{\beta }}}} }[/math]

Step 3: Obtain the confidence bounds on time given the cumulative failure intensity by solving for [math]\displaystyle{ {{t}_{l}} }[/math] and [math]\displaystyle{ {{t}_{u}} }[/math] in the following equations:

[math]\displaystyle{ \begin{align} & {{t}_{l}}= & \frac{\chi _{\tfrac{\alpha }{2},2N}^{2}}{2\cdot {{\lambda }_{c}}(T)} \\ & {{t}_{u}}= & \frac{\chi _{1-\tfrac{\alpha }{2},2N+2}^{2}}{2\cdot {{\lambda }_{c}}(T)} \end{align} }[/math]

Bounds on Time Given Instantaneous Failure Intensity

Fisher Matrix Bounds

These bounds are based on:

[math]\displaystyle{ \frac{\ln (\widehat{T})-\ln (T)}{\sqrt{Var\left[ \ln (\widehat{T}) \right]}}\sim N(0,1) }[/math]

The confidence bounds on the time are given by:

[math]\displaystyle{ CB=\widehat{T}{{e}^{\pm {{z}_{\alpha }}\sqrt{Var(\widehat{T})}/\widehat{T}}} }[/math]

where:

[math]\displaystyle{ \begin{align} & Var(\widehat{T})= & {{\left( \frac{\partial T}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial T}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda }) \\ & & +2\left( \frac{\partial T}{\partial \beta } \right)\left( \frac{\partial T}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda }) \end{align} }[/math]

The variance calculation is the same as Eqns. (var1), (var2) and (var3).

[math]\displaystyle{ \widehat{T}={{\left( \frac{{{\lambda }_{i}}(T)}{\lambda \cdot \beta } \right)}^{1/(\beta -1)}} }[/math]

[math]\displaystyle{ \begin{align} & \frac{\partial T}{\partial \beta }= & {{\left( \frac{{{\lambda }_{i}}(T)}{\lambda \cdot \beta } \right)}^{1/(\beta -1)}}[-\frac{\ln (\tfrac{{{\lambda }_{i}}(T)}{\lambda \cdot \beta })}{{{(\beta -1)}^{2}}}+\frac{1}{\beta (1-\beta )}] \\ & \frac{\partial T}{\partial \lambda }= & {{\left( \frac{{{\lambda }_{i}}(T)}{\lambda \cdot \beta } \right)}^{1/(\beta -1)}}\frac{1}{\lambda (1-\beta )} \end{align} }[/math]

Crow Bounds

Step 1: Calculate [math]\displaystyle{ {{\lambda }_{i}}(T)=\tfrac{1}{MTB{{F}_{i}}} }[/math] .
Step 2: Use the equations from 13.1.7.9 to calculate the bounds on time given the instantaneous failure intensity.

Bounds on Reliability

Fisher Matrix Bounds

These bounds are based on:

[math]\displaystyle{ \log it(\widehat{R}(t))\sim N(0,1) }[/math]

[math]\displaystyle{ \log it(\widehat{R}(t))=\ln \left\{ \frac{\widehat{R}(t)}{1-\widehat{R}(t)} \right\} }[/math]

The confidence bounds on reliability are given by:

[math]\displaystyle{ CB=\frac{\widehat{R}(t)}{\widehat{R}(t)+(1-\widehat{R}(t)){{e}^{\pm {{z}_{\alpha }}\sqrt{Var(\widehat{R}(t))}/\left[ \widehat{R}(t)(1-\widehat{R}(t)) \right]}}} }[/math]

[math]\displaystyle{ Var(\widehat{R}(t))={{\left( \frac{\partial R}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial R}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda })+2\left( \frac{\partial R}{\partial \beta } \right)\left( \frac{\partial R}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda }) }[/math]

The variance calculation is the same as Eqns. (var1), (var2) and (var3).

[math]\displaystyle{ \begin{align} & \frac{\partial R}{\partial \beta }= & {{e}^{-[\widehat{\lambda }{{(t+d)}^{\widehat{\beta }}}-\widehat{\lambda }{{t}^{\widehat{\beta }}}]}}[\lambda {{t}^{\widehat{\beta }}}\ln (t)-\lambda {{(t+d)}^{\widehat{\beta }}}\ln (t+d)] \\ & \frac{\partial R}{\partial \lambda }= & {{e}^{-[\widehat{\lambda }{{(t+d)}^{\widehat{\beta }}}-\widehat{\lambda }{{t}^{\widehat{\beta }}}]}}[{{t}^{\widehat{\beta }}}-{{(t+d)}^{\widehat{\beta }}}] \end{align} }[/math]

Crow Bounds

Failure Terminated Data
With failure terminated data, the 100( [math]\displaystyle{ 1-\alpha }[/math] )% confidence interval for the current reliability at time [math]\displaystyle{ t }[/math] in a specified mission time [math]\displaystyle{ d }[/math] is:

[math]\displaystyle{ ({{[\widehat{R}(d)]}^{\tfrac{1}{{{p}_{1}}}}},{{[\hat{R}(d)]}^{\tfrac{1}{{{p}_{2}}}}}) }[/math]

where

[math]\displaystyle{ \widehat{R}(\tau )={{e}^{-[\widehat{\lambda }{{(t+\tau )}^{\widehat{\beta }}}-\widehat{\lambda }{{t}^{\widehat{\beta }}}]}} }[/math]

[math]\displaystyle{ {{p}_{1}} }[/math] and [math]\displaystyle{ {{p}_{2}} }[/math] can be obtained from Eqn. (ft).

Time Terminated Data
With time terminated data, the 100( [math]\displaystyle{ 1-\alpha }[/math] )% confidence interval for the current reliability at time [math]\displaystyle{ t }[/math] in a specified mission time [math]\displaystyle{ \tau }[/math] is:

[math]\displaystyle{ ({{[\widehat{R}(d)]}^{\tfrac{1}{{{p}_{1}}}}},{{[\hat{R}(d)]}^{\tfrac{1}{{{p}_{2}}}}}) }[/math]

where:

[math]\displaystyle{ \widehat{R}(d)={{e}^{-[\widehat{\lambda }{{(t+d)}^{\widehat{\beta }}}-\widehat{\lambda }{{t}^{\widehat{\beta }}}]}} }[/math]

[math]\displaystyle{ {{p}_{1}} }[/math] and [math]\displaystyle{ {{p}_{2}} }[/math] can be obtained from Eqn. (tt).

Bounds on Time Given Reliability and Mission Time

Fisher Matrix Bounds

The time, [math]\displaystyle{ t }[/math] , must be positive, thus [math]\displaystyle{ \ln t }[/math] is approximately treated as being normally distributed.

[math]\displaystyle{ \frac{\ln (\hat{t})-\ln (t)}{\sqrt{Var\left[ \ln (\hat{t}) \right]}}\sim N(0,1) }[/math]

The confidence bounds on time are calculated by using:

[math]\displaystyle{ CB=\hat{t}{{e}^{\pm {{z}_{\alpha }}\sqrt{Var(\hat{t})}/\hat{t}}} }[/math]

where:

[math]\displaystyle{ Var(\hat{t})={{\left( \frac{\partial t}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial t}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda })+2\left( \frac{\partial t}{\partial \beta } \right)\left( \frac{\partial t}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda }) }[/math]

[math]\displaystyle{ \hat{t} }[/math] is calculated numerically from:

[math]\displaystyle{ \widehat{R}(d)={{e}^{-[\widehat{\lambda }{{(\hat{t}+d)}^{\widehat{\beta }}}-\widehat{\lambda }{{{\hat{t}}}^{\widehat{\beta }}}]}}\text{ };\text{ }d\text{ = mission time} }[/math]

The variance calculations are done by:

[math]\displaystyle{ \begin{align} & \frac{\partial t}{\partial \beta }= & \frac{{{{\hat{t}}}^{{\hat{\beta }}}}\ln (\hat{t})-{{(\hat{t}+d)}^{{\hat{\beta }}}}\ln (\hat{t}+d)}{\hat{\beta }{{(\hat{t}+d)}^{\hat{\beta }-1}}-\hat{\beta }{{{\hat{t}}}^{\hat{\beta }-1}}} \\ & \frac{\partial t}{\partial \lambda }= & \frac{{{{\hat{t}}}^{{\hat{\beta }}}}-{{(\hat{t}+d)}^{{\hat{\beta }}}}}{\hat{\lambda }\hat{\beta }{{(\hat{t}+d)}^{\hat{\beta }-1}}-\hat{\lambda }\hat{\beta }{{{\hat{t}}}^{\hat{\beta }-1}}} \end{align} }[/math]

Crow Bounds

Failure Terminated Data
Step 1: Calculate [math]\displaystyle{ ({{\hat{R}}_{lower}},{{\hat{R}}_{upper}})=({{R}^{\tfrac{1}{{{p}_{1}}}}},{{R}^{\tfrac{1}{{{p}_{2}}}}}) }[/math] .
Step 2: Let [math]\displaystyle{ R={{\hat{R}}_{lower}} }[/math] and solve for [math]\displaystyle{ {{t}_{1}} }[/math] numerically using [math]\displaystyle{ R={{e}^{-[\widehat{\lambda }{{({{{\hat{t}}}_{1}}+d)}^{\widehat{\beta }}}-\widehat{\lambda }\hat{t}_{1}^{\widehat{\beta }}]}} }[/math] .
Step 3: Let [math]\displaystyle{ R={{\hat{R}}_{upper}} }[/math] and solve for [math]\displaystyle{ {{t}_{2}} }[/math] numerically using [math]\displaystyle{ R={{e}^{-[\widehat{\lambda }{{({{{\hat{t}}}_{2}}+d)}^{\widehat{\beta }}}-\widehat{\lambda }\hat{t}_{2}^{\widehat{\beta }}]}} }[/math] .
Step 4: If [math]\displaystyle{ {{t}_{1}}\lt {{t}_{2}} }[/math] , then [math]\displaystyle{ {{t}_{lower}}={{t}_{1}} }[/math] and [math]\displaystyle{ {{t}_{upper}}={{t}_{2}} }[/math] . If [math]\displaystyle{ {{t}_{1}}\gt {{t}_{2}} }[/math] , then [math]\displaystyle{ {{t}_{lower}}={{t}_{2}} }[/math] and [math]\displaystyle{ {{t}_{upper}}={{t}_{1}} }[/math] .

Time Terminated Data
Step 1: Calculate [math]\displaystyle{ ({{\hat{R}}_{lower}},{{\hat{R}}_{upper}})=({{R}^{\tfrac{1}{{{\Pi }_{1}}}}},{{R}^{\tfrac{1}{{{\Pi }_{2}}}}}) }[/math] .
Step 2: Let [math]\displaystyle{ R={{\hat{R}}_{lower}} }[/math] and solve for [math]\displaystyle{ {{t}_{1}} }[/math] numerically using [math]\displaystyle{ R={{e}^{-[\widehat{\lambda }{{({{{\hat{t}}}_{1}}+d)}^{\widehat{\beta }}}-\widehat{\lambda }\hat{t}_{1}^{\widehat{\beta }}]}} }[/math] .
Step 3: Let [math]\displaystyle{ R={{\hat{R}}_{upper}} }[/math] and solve for [math]\displaystyle{ {{t}_{2}} }[/math] numerically using [math]\displaystyle{ R={{e}^{-[\widehat{\lambda }{{({{{\hat{t}}}_{2}}+d)}^{\widehat{\beta }}}-\widehat{\lambda }\hat{t}_{2}^{\widehat{\beta }}]}} }[/math] .
Step 4: If [math]\displaystyle{ {{t}_{1}}\lt {{t}_{2}} }[/math] , then [math]\displaystyle{ {{t}_{lower}}={{t}_{1}} }[/math] and [math]\displaystyle{ {{t}_{upper}}={{t}_{2}} }[/math] . If [math]\displaystyle{ {{t}_{1}}\gt {{t}_{2}} }[/math] , then [math]\displaystyle{ {{t}_{lower}}={{t}_{2}} }[/math] and [math]\displaystyle{ {{t}_{upper}}={{t}_{1}} }[/math] .

Bounds on Mission Time Given Reliability and Time

Fisher Matrix Bounds

The mission time, [math]\displaystyle{ d }[/math] , must be positive, thus [math]\displaystyle{ \ln \left( d \right) }[/math] is approximately treated as being normally distributed.

[math]\displaystyle{ \frac{\ln (\hat{d})-\ln (d)}{\sqrt{Var\left[ \ln (\hat{d}) \right]}}\sim N(0,1) }[/math]

The confidence bounds on mission time are given by using:

[math]\displaystyle{ CB=\hat{d}{{e}^{\pm {{z}_{\alpha }}\sqrt{Var(\hat{d})}/\hat{d}}} }[/math]

where:

[math]\displaystyle{ Var(\hat{d})={{\left( \frac{\partial d}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial d}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda })+2\left( \frac{\partial td}{\partial \beta } \right)\left( \frac{\partial d}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda }) }[/math]

Calculate [math]\displaystyle{ \hat{d} }[/math] from:

[math]\displaystyle{ \hat{d}={{\left[ {{t}^{{\hat{\beta }}}}-\frac{\ln (R)}{{\hat{\lambda }}} \right]}^{\tfrac{1}{{\hat{\beta }}}}}-t }[/math]

The variance calculations are done by:

[math]\displaystyle{ \begin{align} & \frac{\partial d}{\partial \beta }= & \left[ \frac{{{t}^{{\hat{\beta }}}}\ln (t)}{{{(t+\hat{d})}^{{\hat{\beta }}}}}-\ln (t+\hat{d}) \right]\cdot \frac{t+\hat{d}}{{\hat{\beta }}} \\ & \frac{\partial d}{\partial \lambda }= & \frac{{{t}^{{\hat{\beta }}}}-{{(t+\hat{d})}^{{\hat{\beta }}}}}{\hat{\lambda }\hat{\beta }{{(t+\hat{d})}^{\hat{\beta }-1}}} \end{align} }[/math]

Crow Bounds

Failure Terminated Data
Step 1: Calculate [math]\displaystyle{ ({{\hat{R}}_{lower}},{{\hat{R}}_{upper}})=({{R}^{\tfrac{1}{{{p}_{1}}}}},{{R}^{\tfrac{1}{{{p}_{2}}}}}) }[/math] .
Step 2: Let [math]\displaystyle{ R={{\hat{R}}_{lower}} }[/math] and solve for [math]\displaystyle{ {{d}_{1}} }[/math] such that:

[math]\displaystyle{ {{d}_{1}}={{\left( {{t}^{{\hat{\beta }}}}-\frac{\ln ({{R}_{lower}})}{{\hat{\lambda }}} \right)}^{\tfrac{1}{{\hat{\beta }}}}}-t }[/math]

Step 3: Let [math]\displaystyle{ R={{\hat{R}}_{upper}} }[/math] and solve for [math]\displaystyle{ {{d}_{2}} }[/math] such that:

[math]\displaystyle{ {{d}_{2}}={{\left( {{t}^{{\hat{\beta }}}}-\frac{\ln ({{R}_{upper}})}{{\hat{\lambda }}} \right)}^{\tfrac{1}{{\hat{\beta }}}}}-t }[/math]

Step 4: If [math]\displaystyle{ {{d}_{1}}\lt {{d}_{2}} }[/math] , then [math]\displaystyle{ {{d}_{lower}}={{d}_{1}} }[/math] and [math]\displaystyle{ {{d}_{upper}}={{d}_{2}} }[/math] . If [math]\displaystyle{ {{d}_{1}}\gt {{d}_{2}} }[/math] , then [math]\displaystyle{ {{d}_{lower}}={{d}_{2}} }[/math] and [math]\displaystyle{ {{d}_{upper}}={{d}_{1}} }[/math] .

Time Terminated Data
Step 1: Calculate [math]\displaystyle{ ({{\hat{R}}_{lower}},{{\hat{R}}_{upper}})=({{R}^{\tfrac{1}{{{\Pi }_{1}}}}},{{R}^{\tfrac{1}{{{\Pi }_{2}}}}}) }[/math] .
Step 2: Let [math]\displaystyle{ R={{\hat{R}}_{lower}} }[/math] and solve for [math]\displaystyle{ {{d}_{1}} }[/math] using Eqn. (CBR1).
Step 3: Let [math]\displaystyle{ R={{\hat{R}}_{upper}} }[/math] and solve for [math]\displaystyle{ {{d}_{2}} }[/math] using Eqn. (CBR2).
Step 4: If [math]\displaystyle{ {{d}_{1}}\lt {{d}_{2}} }[/math] , then [math]\displaystyle{ {{d}_{lower}}={{d}_{1}} }[/math] and [math]\displaystyle{ {{d}_{upper}}={{d}_{2}} }[/math] . If [math]\displaystyle{ {{d}_{1}}\gt {{d}_{2}} }[/math] , then [math]\displaystyle{ {{d}_{lower}}={{d}_{2}} }[/math] and [math]\displaystyle{ {{d}_{upper}}={{d}_{1}} }[/math] .

Bounds on Cumulative Number of Failures

Fisher Matrix Bounds

The cumulative number of failures, [math]\displaystyle{ N(t) }[/math] , must be positive, thus [math]\displaystyle{ \ln \left( N(t) \right) }[/math] is approximately treated as being normally distributed.

[math]\displaystyle{ \frac{\ln (\widehat{N}(t))-\ln (N(t))}{\sqrt{Var\left[ \ln \widehat{N}(t) \right]}}\sim N(0,1) }[/math]

[math]\displaystyle{ N(t)=\widehat{N}(t){{e}^{\pm {{z}_{\alpha }}\sqrt{Var(\widehat{N}(t))}/\widehat{N}(t)}} }[/math]

where:

[math]\displaystyle{ \widehat{N}(t)=\widehat{\lambda }{{t}^{\widehat{\beta }}} }[/math]

[math]\displaystyle{ \begin{align} & Var(\widehat{N}(t))= & {{\left( \frac{\partial N(t)}{\partial \beta } \right)}^{2}}Var(\widehat{\beta })+{{\left( \frac{\partial N(t)}{\partial \lambda } \right)}^{2}}Var(\widehat{\lambda }) \\ & & +2\left( \frac{\partial N(t)}{\partial \beta } \right)\left( \frac{\partial N(t)}{\partial \lambda } \right)cov(\widehat{\beta },\widehat{\lambda }) \end{align} }[/math]

The variance calculation is the same as Eqns. (var1), (var2) and (var3).

[math]\displaystyle{ \begin{align} & \frac{\partial N(t)}{\partial \beta }= & \hat{\lambda }{{t}^{\widehat{\beta }}}\ln (t) \\ & \frac{\partial N(t)}{\partial \lambda }= & t\widehat{\beta } \end{align} }[/math]

Crow Bounds

[math]\displaystyle{ \begin{array}{*{35}{l}} {{N}_{L}}(T)=\tfrac{T}{\widehat{\beta }}{{\lambda }_{i}}{{(T)}_{L}} \\ {{N}_{U}}(T)=\tfrac{T}{\widehat{\beta }}{{\lambda }_{i}}{{(T)}_{U}} \\ \end{array} }[/math]

where [math]\displaystyle{ {{\lambda }_{i}}{{(T)}_{L}} }[/math] and [math]\displaystyle{ {{\lambda }_{i}}{{(T)}_{U}} }[/math] can be obtained from Eqn. (inr).

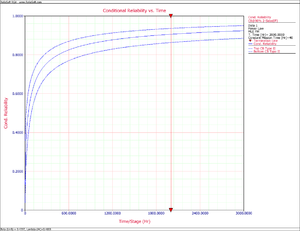
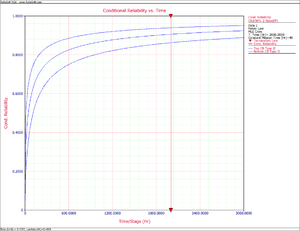
Example 3

Using the data from Example 1, calculate the mission reliability at [math]\displaystyle{ t=2000 }[/math] hours and mission time [math]\displaystyle{ d=40 }[/math] hours along with the confidence bounds at the 90% confidence level.
Solution
The maximum likelihood estimates of [math]\displaystyle{ \widehat{\lambda } }[/math] and [math]\displaystyle{ \widehat{\beta } }[/math] from Example 1 are:

[math]\displaystyle{ \begin{align} & \widehat{\beta }= & 0.45300 \\ & \widehat{\lambda }= & 0.36224 \end{align} }[/math]

From Eq. (reliability), the mission reliability at [math]\displaystyle{ t=2000 }[/math] for mission time [math]\displaystyle{ d=40 }[/math] is:

[math]\displaystyle{ \begin{align} & \widehat{R}(t)= & {{e}^{-\left[ \lambda {{\left( t+d \right)}^{\beta }}-\lambda {{t}^{\beta }} \right]}} \\ & = & 0.90292 \end{align} }[/math]

At the 90% confidence level and [math]\displaystyle{ T=2000 }[/math] hours, the Fisher Matrix confidence bounds for the mission reliability for mission time [math]\displaystyle{ d=40 }[/math] are given by:

[math]\displaystyle{ CB=\frac{\widehat{R}(t)}{\widehat{R}(t)+(1-\widehat{R}(t)){{e}^{\pm {{z}_{\alpha }}\sqrt{Var(\widehat{R}(t))}/\left[ \widehat{R}(t)(1-\widehat{R}(t)) \right]}}} }[/math]

[math]\displaystyle{ \begin{align} & {{[\widehat{R}(t)]}_{L}}= & 0.83711 \\ & {{[\widehat{R}(t)]}_{U}}= & 0.94392 \end{align} }[/math]

The Crow confidence bounds for the mission reliability are:

[math]\displaystyle{ \begin{align} & {{[\widehat{R}(t)]}_{L}}= & {{[\widehat{R}(\tau )]}^{\tfrac{1}{{{\Pi }_{1}}}}} \\ & = & {{[0.90292]}^{\tfrac{1}{0.71440}}} \\ & = & 0.86680 \\ & {{[\widehat{R}(t)]}_{U}}= & {{[\widehat{R}(\tau )]}^{\tfrac{1}{{{\Pi }_{2}}}}} \\ & = & {{[0.90292]}^{\tfrac{1}{1.6051}}} \\ & = & 0.93836 \end{align} }[/math]

Figures ConfReliFish and ConfRelCrow show the Fisher Matrix and Crow confidence bounds on mission reliability for mission time [math]\displaystyle{ d=40 }[/math] .

Conditional Reliability vs. Time plot with Fisher Matrix confidence bounds.

Conditional Reliability vs. Time plot with Crow confidence bounds.