Test-Fix-Test Data Reference Example

New format available! This reference is now available in a new format that offers faster page load, improved display for calculations and images and more targeted search.

As of January 2024, this Reliawiki page will not continue to be updated. Please update all links and bookmarks to the latest references at RGA examples and RGA reference examples.

Test-Fix-Test Data

This example compares the results for test-fix-test data (time terminated).

Reference Case

Crow, L.H., Confidence Interval Procedures for the Weibull Process with Applications to Reliability Growth, U.S. Army Material Systems Analysis Activity, 1981.

For this example, the following will be calculated:

Parameters of the Crow-AMSAA (NHPP) model
Demonstrated MTBF (DMTBF)
80% two-sided confidence bounds on the DMTBF

Data

The following table shows the data.

**Termination Time = 500 hours**
0.2
4.2
4.5
5
5.4
6.1
7.9
14.8
19.2
48.6
85.8
108.9
127.2
129.8
150.1
159.7
227.4
244.7
262.7
315.3
329.6
404.3
486.2

Result

The book has the following results:

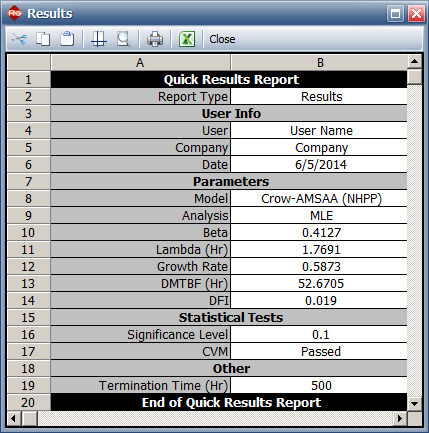
Beta = 0.413, Lambda = 1.769

DMTBF = 52.7

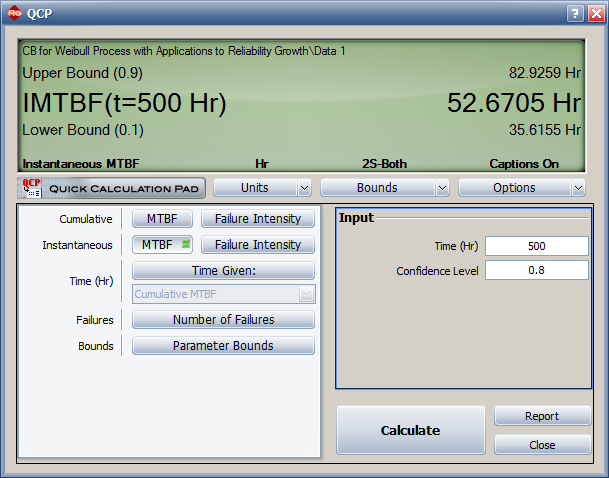
Confidence Bounds on DMTBF (CL = 80%) = (35.6, 82.9)

Results in RGA

In RGA, the Crow-AMSAA (NHPP) model with the maximum likelihood estimation (MLE) method was used to calculate the results. The following equations are used to calculate [math]\displaystyle{ \beta\,\! }[/math] and [math]\displaystyle{ \lambda\,\! }[/math].

[math]\displaystyle{ \begin{align} \hat{\beta }=&\frac{N}{N\ln T^{*}-\underset{i=1}{\overset{N}{\mathop \sum }}\,T_{i}}\\ \\ =&\frac{23}{{23\cdot \ln \left ( 500 \right )}-87.2106}\\ \\ =&0.4127 \end{align}\,\! }[/math]

[math]\displaystyle{ \begin{align} \hat{\lambda }=&\frac{N}{T^{*\beta }}\\ \\ =&\frac{23}{500^{0.4127}}\\ \\ =&1.7691 \end{align}\,\! }[/math]

The model parameters are:

The instantaneous MTBF and its two-sided 80% confidence bounds are:

Test-Fix-Test Data Reference Example

Navigation menu

Search