This example compares the results from the statistically optimum test plan in the ALTA test plan tool for two stresses.
Reference Case
The data set is from Example 20.8 on page 549 in the book Statistical Methods for Reliability Data by Dr. Meeker and Dr. Escobar, John Wiley & Sons, 1998.
Data
A Weibull distribution with the following life stress relationship is used.
- [math]\displaystyle{ ln(\eta)=\beta_{0}+\beta_{1} \times log(vpm) + \beta_{2} \frac{11605}{temp}\,\! }[/math]
where temp is the temperature in K and vpm is the voltage stress in volts/mm. The planning values for the model parameters used in the test plan are:
- [math]\displaystyle{ \beta = \frac{1}{\sigma} = 1.485\,\! }[/math] , [math]\displaystyle{ \beta_{1} = 12.28\,\! }[/math] and [math]\displaystyle{ \beta_{2} = 0.3878\,\! }[/math]
These planning values are given on page 535.
The use condition stress values are vpm = 80 volts/mm and temp = 120°C (393.15°K).
The highest stress values that can be used in the test are vpm = 200 volts/mm and temp = 260°C (533.15°K).
A total of 170 units are available for testing for 1,000 hours. Three combinations of temperature and voltage levels can be used in the test. The objective of the test plan is to:
- Determine the three stress combinations used in the test.
- Determine the number of test units at each stress combination.
Tests will be conducted using the created test plan. The failure data from the test will be used to estimate the model parameters. The estimated parameters are then used to predict the B10 life at vpm = 80 volts/mm and temp = 120°C.
We need to find a test plan that will minimize the estimation variance of the B10 life at the given usage stress level.
Result
- The three stress combinations and the number of test units at each of them are:
- 62 units will be tested at voltage of 124 and temperature of 260°C (533.15°K)
- 42 units will be tested at voltage of 159 and temperature of 120°C (393.15°K)
- 66 units will be tested at voltage of 200 and temperature of 260°C (533.15°K)
- The estimated standard error for the B10 life at vpm = 80 volts/mm and temp = 120°C from this test plan is [math]\displaystyle{ Ase \left[log(\hat{t}_{0.1}(50))\right]\,\! }[/math] = 0.3670 .
The standard error is calculated from the result in Table 20.6. In Table 20.6, the value for [math]\displaystyle{ \frac{n}{\sigma^{2}}Var\left[log(\hat{t}_{0.1}(50)) \right]\,\! }[/math] = 50.5. Since n = 170 and [math]\displaystyle{ \frac{1}{\sigma^{2}} = \beta^{2}\,\! }[/math] = 2.205229, so [math]\displaystyle{ Var\left [log(\hat{t}_{0.1}(50)) \right]\,\! }[/math] = 0.1347. Therefore, [math]\displaystyle{ Ase \left[log(\hat{t}_{0.1}(50)) \right]\,\! }[/math] = 0.3670.
Results in ALTA
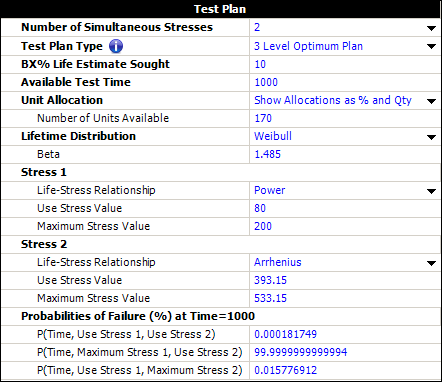
The planning information is entered in ALTA, as shown below.
The probabilities of failure (%) at time of 1000 are calculated based on test planning values. For example, at the use stress of voltage and at the maximum stress of temperature, the life characteristic is:
- [math]\displaystyle{ \begin{align}
ln(\eta) =& \beta_{0}+\beta_{1} \times log(vpm) + \beta_{2} \frac{11605}{temp} \\
=& 58.173 - 12.28 \times ln(80) + 0.3878 \frac{11605}{533.15}\\
=& 12.8029
\end{align}\,\! }[/math]
Therefore, [math]\displaystyle{ \eta\,\! }[/math] = 363269. The probability of failure is calculated by:
- [math]\displaystyle{ \begin{align}
P(Time, Use Stress 1, Maximum Stress 2) =& P(1000, 80, 533.15)\\
=& 1 - e^{-\left(\frac{1000}{363269} \right)^{1.485}}\\
=& 0.000158 = 0.0158%
\end{align}\,\! }[/math]
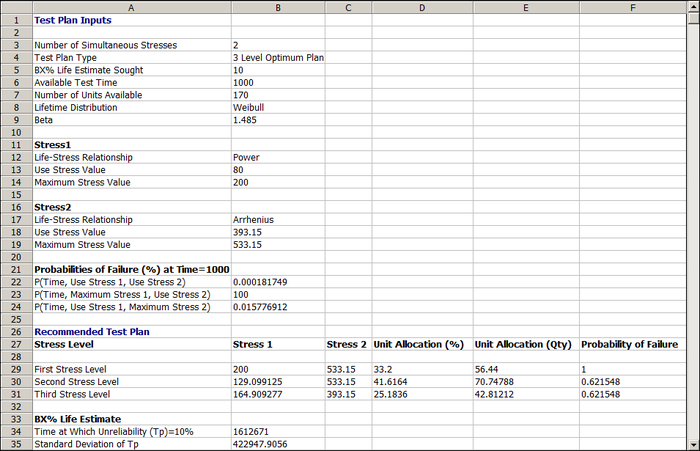
The three level best compromise test plan in ALTA is shown below.
The test plan shows that:
- The first stress level (row 29) should be a combination of 200 volts and 260°C (533.15°K) and about 56 units should be tested at that level.
- The second stress level (row 30) should be a combination of 129 volts and 260°C (533.15°K) and about 71 units should be tested at that level.
- The third stress level (row 31) should be a combination of 165 volts and 120°C (393.15°K) and about 43 units should be tested at that level.
The estimated standard deviation of the log B10 life at 50°C can be obtained by:
- [math]\displaystyle{ Ase \left[ log \left(\hat{t}_{0.1}(50) \right)\right] = \frac{Ase\left(\hat{t}_{0.1}(50) \right)}{\hat{t}_{0.1}(50)} = \frac{422947.9056}{1612671} = 0.262265\,\! }[/math]
The above results are different from the results given in the book. In the following discussion, a simulation study is conducted to check the results obtained from ALTA.
Using ALTA SimuMatic to Evaluate the Test Plan
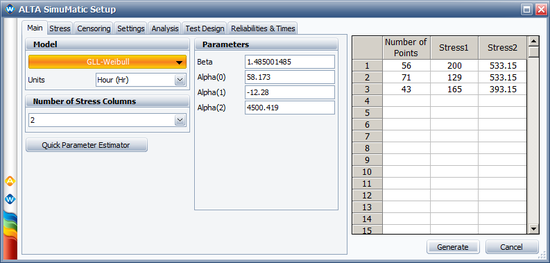
We will use the SimuMatic utility to evaluate the test plan. The following picture shows the settings used in the Main tab of SimuMatic (with [math]\displaystyle{ \alpha_{1} = \beta_{1} \times 11605\,\! }[/math]):
The rest of the SimuMatic settings are as follows:
- Use level = 80, Transformation = Logarithmic
- Use level = 393.15, Transformation = Reciprocal
- Right censoring after time 1000.
- Seed = 10, Precision = 4, Number of data sets = 1000
- Reliability value = 0.9
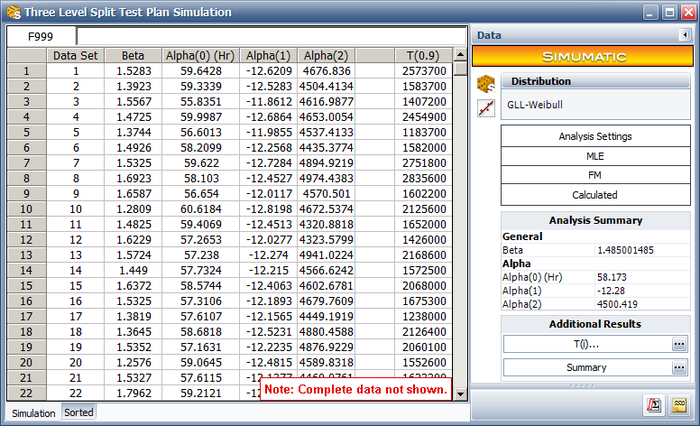
The simulation results are shown next.
We take the nature log of the B10 life (the last column) and calculate its standard deviation. The calculated standard deviation is 0.2629. This is very close to the analytical solution from the ALTA test plan tool, where the standard deviation is 0.2623.
This standard deviation is smaller than the standard deviation given by the test plan in the book. Therefore, the test plan generated in ALTA is better than the test plan given in the book in terms of minimizing the estimation variance of the B10 life.