Template:Example: Test Design Using Expected Failure Times Plot: Difference between revisions
No edit summary |
No edit summary |
||
| Line 10: | Line 10: | ||
[[Image: Expected Failure Plot example Result Summary.png|thumb|center|400px]] | [[Image: Expected Failure Plot example Result Summary.png|thumb|center|400px]] | ||
[[Image: Expected Failure Plot example Result Plot.png|thumb|center|400px]] | [[Image: Expected Failure Plot example Result Plot.png|thumb|center|400px]] | ||
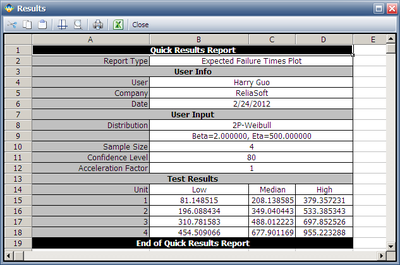
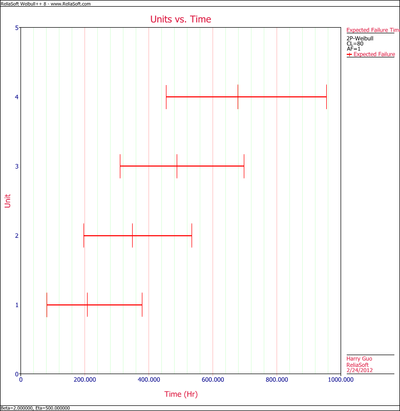
From the above results, we can see the upper bound of the last failure is about 955 hours. Therefore, the test probably will last for around 955 hours. | |||
As we know, with four samples, the median rank for the second failure is 0.385728. Using this value and the assumed Weibull distribution, the median value of the failure time of the 2nd failure is calcualted as: | |||
<math>\begin{align} | |||
& Q=1-{{e}^{{{\left( \frac{t}{\eta } \right)}^{\beta }}}}\Rightarrow \\ | |||
& \ln (1-Q)={{\left( \frac{t}{\eta } \right)}^{\beta }} \\ | |||
& \Rightarrow t=\text{349.04}\\ | |||
\end{align}</math> | |||
Its bounds and other failure times can be calcualted in a similar way. | |||
Revision as of 18:00, 24 February 2012
Test Design Using Expected Failure Times Plot
Four units were allocated for a reliability test. The test engineers want to know how long the test will last if all the units are tested to failure. Based on previous experiments, they assume the underlying failure distribution is a Weibull distribution with [math]\displaystyle{ \beta }[/math] = 2 and [math]\displaystyle{ \eta }[/math] = 500.
Solution
The expected failure time with 80% 2-sided confidence bounds are given below.
From the above results, we can see the upper bound of the last failure is about 955 hours. Therefore, the test probably will last for around 955 hours.
As we know, with four samples, the median rank for the second failure is 0.385728. Using this value and the assumed Weibull distribution, the median value of the failure time of the 2nd failure is calcualted as:
[math]\displaystyle{ \begin{align} & Q=1-{{e}^{{{\left( \frac{t}{\eta } \right)}^{\beta }}}}\Rightarrow \\ & \ln (1-Q)={{\left( \frac{t}{\eta } \right)}^{\beta }} \\ & \Rightarrow t=\text{349.04}\\ \end{align} }[/math]
Its bounds and other failure times can be calcualted in a similar way.