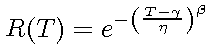Weibull++ Standard Folio Data 1P-Weibull: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
No edit summary |
||
| Line 28: | Line 28: | ||
[[Image:Docedit.png|right|20px|link=http://www.reliawiki.com/index.php?title=Weibull%2B%2B_Standard_Folio_Data_1P-Weibull&action=edit]] | [[Image:Docedit.png|right|20px|link=http://www.reliawiki.com/index.php?title=Weibull%2B%2B_Standard_Folio_Data_1P-Weibull&action=edit]] | ||
[[Category:Needs example]] | [[Category:Needs example]] | ||
Revision as of 19:14, 8 February 2012
The One-Parameter Weibull DistributionThe one-parameter Weibull reliability function is obtained by again setting [math]\displaystyle{ \gamma=0 \,\! }[/math] and assuming [math]\displaystyle{ \beta=C=Constant \,\! }[/math] assumed value or: where the only unknown parameter is the scale parameter, [math]\displaystyle{ \eta\,\! }[/math]. Note that in the formulation of the one-parameter Weibull, we assume that the shape parameter [math]\displaystyle{ \beta \,\! }[/math] is known a priori from past experience on identical or similar products. The advantage of doing this is that data sets with few or no failures can be analyzed.
|
| The Weibull Distribution |