Stress-Strength Analysis in Design for Reliability: Difference between revisions

From ReliaWiki
Jump to navigation Jump to search
No edit summary
No edit summary
Line 1: Line 1:
'''Determining the Strength Distribution for a Stress-Strength Analysis'''
'''Using Stress-Strength Analysis to Determine the Required Strength Distribution'''


Assume the stress distribution for a component is known and it is a Weibull distribution with beta=3 and eta=2000. For the current design, the strength distribution is also a Weibull distribution with beta =1.5 and eta=4000.
Assume the stress distribution for a component is known and it is a Weibull distribution with beta=3 and eta=2000. For the current design, the strength distribution is also a Weibull distribution with beta =1.5 and eta=4000.


:*Evaluate the current reliability.  
:*Evaluate the current reliability.  
:*If the reliability does not meet the target reliability of 90%, please use the Target Reliability Parameter Estimator to determine what parameters would be required for the strength distribution in order to meet the specified target.
:*If the reliability does not meet the target reliability of 90%, use the Target Reliability Parameter Estimator to determine what parameters would be required for the strength distribution in order to meet the specified target.




'''Solution'''
'''Solution'''


First, we need create two empty Weibull++ standard folio and enter the distribution parameters for the stress and strenght distribution. For example, for the stress distribution, it is:
First, we need create two empty Weibull++ standard folios and enter the distribution parameters for the stress and strength distributions. For example, for the stress distribution, it is:


[[Image: Stress Distribution Example 2.png|thumb|center|400px]]
[[Image: Stress Distribution Example 2.png|thumb|center|400px]]
Line 18: Line 18:
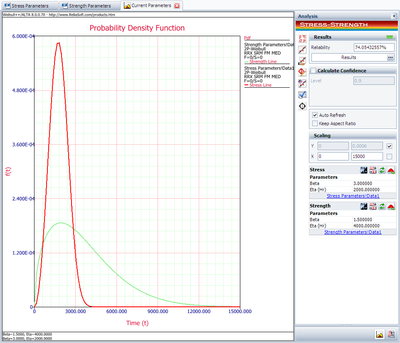
[[Image: Stress-strength example 2 current reliability.png|thumb|center|400px]]
[[Image: Stress-strength example 2 current reliability.png|thumb|center|400px]]


The above result shows that the current reliability is about 74.05% which is below the target value of 90%. We need to use the '''Target Reliability Parameter Estimator''' to determine the distribution parameter for the strength distribution. Click on the following icon:
The above result shows that the current reliability is about 74.05%, which is below the target value of 90%. We need to use the '''Target Reliability Parameter Estimator''' to determine the parameter for the strength distribution that would be required to meet the target. Click on the following icon:


[[Image:Stress-strength example 2 target R icon.png|thumb|center|400px]]
[[Image:Stress-strength example 2 target R icon.png|thumb|center|400px]]




Choose to calculate for Eta under '''Strength Parameters''', set the Target Reliability to 90%, and click '''Calculate''':
Choose to calculate for eta under '''Strength Parameters''', set the Target Reliability to 90%, and click '''Calculate''':


[[Image:Stress-strength example 2 Result.png|thumb|center|400px]]
[[Image:Stress-strength example 2 Result.png|thumb|center|400px]]




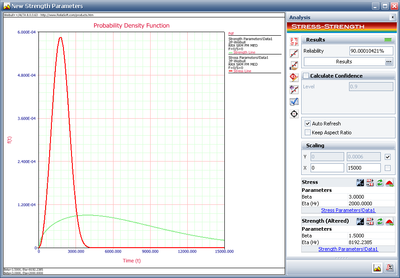
The calculated eta is 8192.2385. Click '''Update''' to perform the stress-strength analysis again using the altered parameters for the strength distribution.
The calculated eta is 8192.2385 hours. Click '''Update''' to perform the stress-strength analysis again using the altered parameters for the strength distribution.


The plot shows the calculated reliability indeed is 90%.  
The plot shows the calculated reliability indeed is 90%.  
Line 35: Line 35:




Therefore, in order to meet the reliability requirement, the component must to be redesigned with the eta to be at least of 8192.2385.
Therefore, in order to meet the reliability requirement, the component must be redesigned with the eta to be at least 8192.2385 hours.

Revision as of 18:42, 30 March 2012

Using Stress-Strength Analysis to Determine the Required Strength Distribution

Assume the stress distribution for a component is known and it is a Weibull distribution with beta=3 and eta=2000. For the current design, the strength distribution is also a Weibull distribution with beta =1.5 and eta=4000.

  • Evaluate the current reliability.
  • If the reliability does not meet the target reliability of 90%, use the Target Reliability Parameter Estimator to determine what parameters would be required for the strength distribution in order to meet the specified target.


Solution

First, we need create two empty Weibull++ standard folios and enter the distribution parameters for the stress and strength distributions. For example, for the stress distribution, it is:

Stress Distribution Example 2.png


Then, following the steps given in Example 1, the reliability of the current design is given in the following figure:

Stress-strength example 2 current reliability.png

The above result shows that the current reliability is about 74.05%, which is below the target value of 90%. We need to use the Target Reliability Parameter Estimator to determine the parameter for the strength distribution that would be required to meet the target. Click on the following icon:

Stress-strength example 2 target R icon.png


Choose to calculate for eta under Strength Parameters, set the Target Reliability to 90%, and click Calculate:

Stress-strength example 2 Result.png


The calculated eta is 8192.2385 hours. Click Update to perform the stress-strength analysis again using the altered parameters for the strength distribution.

The plot shows the calculated reliability indeed is 90%.

Stress-strength example 2 Confirmed Result.png


Therefore, in order to meet the reliability requirement, the component must be redesigned with the eta to be at least 8192.2385 hours.

Retrieved from "https://www.reliawiki.com/index.php?title=Stress-Strength_Analysis_in_Design_for_Reliability&oldid=23219"