IPL-Weibull Example: Difference between revisions
Jump to navigation
Jump to search
Nikki Helms (talk | contribs) (Created page with '{| class="FCK__ShowTableBorders" border="0" cellspacing="0" cellpadding="0" align="center"; style="width:100%;" |- | valign="middle" align="left" bgcolor=EEEDF7|[[Image: ALTA-Exa…') |
Nikki Helms (talk | contribs) No edit summary |
||
| Line 3: | Line 3: | ||
| valign="middle" align="left" bgcolor=EEEDF7|[[Image: ALTA-Examples-banner.png|400px|center]] | | valign="middle" align="left" bgcolor=EEEDF7|[[Image: ALTA-Examples-banner.png|400px|center]] | ||
|} | |} | ||
<br> | |||
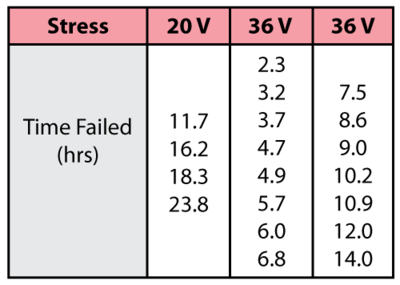
Consider the following times-to-failure data at two different stress levels. | |||
<br> | |||
<br> | |||
[[Image:chp8ex1table.png|center|400px|''Pdf'' of the lognormal distribution with different log-std values.]] | |||
<br> | |||
<br> | |||
The data set was analyzed jointly and with a complete MLE solution over the entire data set using ReliaSoft's ALTA. The analysis yields: | |||
<br> | |||
::<math>\widehat{\beta }=2.616464</math> | |||
<br> | |||
::<math>\widehat{K}=0.001022</math> | |||
<br> | |||
::<math>\widehat{n}=1.327292</math> | |||
Revision as of 21:32, 21 March 2012
Consider the following times-to-failure data at two different stress levels.
The data set was analyzed jointly and with a complete MLE solution over the entire data set using ReliaSoft's ALTA. The analysis yields:
- [math]\displaystyle{ \widehat{\beta }=2.616464 }[/math]
- [math]\displaystyle{ \widehat{K}=0.001022 }[/math]
- [math]\displaystyle{ \widehat{n}=1.327292 }[/math]