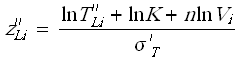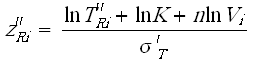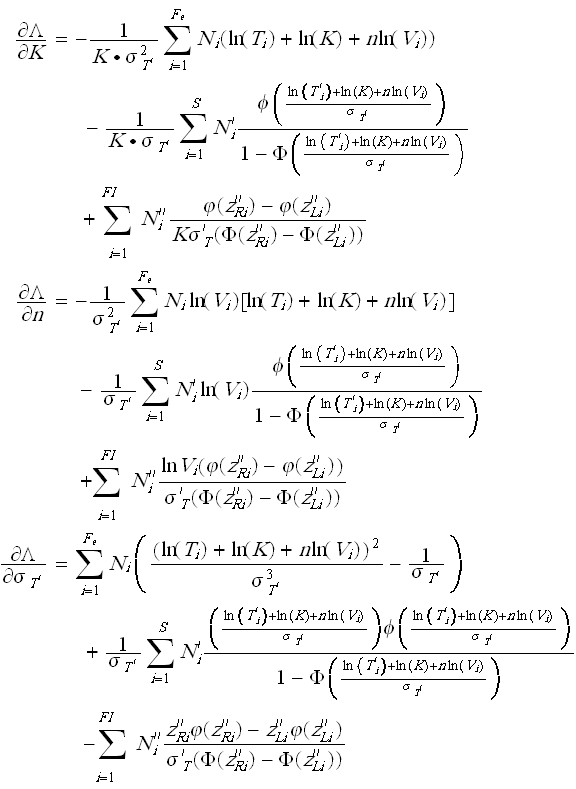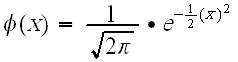Template:Ipl lognormal: Difference between revisions
No edit summary |
No edit summary |
||
| Line 20: | Line 20: | ||
The median of the lognormal distribution is given by: | The median of the lognormal distribution is given by: | ||
<math>\breve{T}=e^{\overline{T}'}</math>(7) | ::<math>\breve{T}=e^{\overline{T}'}</math>(7) | ||
| Line 26: | Line 26: | ||
<math> \breve{T}=L(V)=\frac{1}{K*V^n}</math> | ::<math> \breve{T}=L(V)=\frac{1}{K*V^n}</math> | ||
or: | or: | ||
<math>e^{\overline{T'}}=\frac{1}{K*V^n}</math> | ::<math>e^{\overline{T'}}=\frac{1}{K*V^n}</math> | ||
Thus: | Thus: | ||
<math>\overline{T}'=-ln(K)-n ln(V) </math>(8) | ::<math>\overline{T}'=-ln(K)-n ln(V) </math>(8) | ||
Substituting Eqn. (8) into Eqn. (6) yields the IPL- lognormal model pdf or: | Substituting Eqn. (8) into Eqn. (6) yields the IPL- lognormal model pdf or: | ||
| Line 41: | Line 41: | ||
IPL-Lognormal Statistical Properties Summary | ====IPL-Lognormal Statistical Properties Summary==== | ||
The Mean | ====The Mean==== | ||
The mean life of the IPL-lognormal model (mean of the times-to-failure), , is given by: | The mean life of the IPL-lognormal model (mean of the times-to-failure), , is given by: | ||
| Line 53: | Line 53: | ||
The Standard Deviation | ====The Standard Deviation==== | ||
The standard deviation of the IPL-lognormal model (standard deviation of the times-to-failure), , is given by: | The standard deviation of the IPL-lognormal model (standard deviation of the times-to-failure), , is given by: | ||
| Line 63: | Line 63: | ||
The Mode | ====The Mode==== | ||
The mode of the IPL-lognormal model is given by: | The mode of the IPL-lognormal model is given by: | ||
| Line 69: | Line 69: | ||
IPL-Lognormal Reliability | ====IPL-Lognormal Reliability==== | ||
The reliability for a mission of time T, starting at age 0, for the IPL-lognormal model is determined by: | The reliability for a mission of time T, starting at age 0, for the IPL-lognormal model is determined by: | ||
| Line 79: | Line 79: | ||
Reliable Life | ====Reliable Life==== | ||
The reliable life, or the mission duration for a desired reliability goal, tR is estimated by first solving the reliability equation with respect to time, as follows: | The reliable life, or the mission duration for a desired reliability goal, tR is estimated by first solving the reliability equation with respect to time, as follows: | ||
| Line 97: | Line 97: | ||
Lognormal Failure Rate | ====Lognormal Failure Rate==== | ||
The lognormal failure rate is given by: | The lognormal failure rate is given by: | ||
| Line 103: | Line 103: | ||
Parameter Estimation | ===Parameter Estimation=== | ||
Maximum Likelihood Estimation Method | ====Maximum Likelihood Estimation Method==== | ||
The complete IPL-lognormal log-likelihood function is: | The complete IPL-lognormal log-likelihood function is: | ||
| Line 119: | Line 119: | ||
and: | and: | ||
Fe is the number of groups of exact times-to-failure data points. | |||
Ni is the number of times-to-failure data points in the ith time-to-failure data group. | * Fe is the number of groups of exact times-to-failure data points. | ||
K is the IPL parameter (unknown, the second of three parameters to be estimated). | * Ni is the number of times-to-failure data points in the ith time-to-failure data group. | ||
n is the second IPL parameter (unknown, the third of three parameters to be estimated). | |||
Vi is the stress level of the ith group. | * <math>s_{T'}</math> is the standard deviation of the natural logarithm of the times-to-failure (unknown, the first of three parameters to be estimated). | ||
Ti is the exact failure time of the ith group. | |||
S is the number of groups of suspension data points. | * <math>K</math> is the IPL parameter (unknown, the second of three parameters to be estimated). | ||
* <math>n</math> is the second IPL parameter (unknown, the third of three parameters to be estimated). | |||
* <math>Vi</math> is the stress level of the ith group. | |||
* <math>Ti</math> is the exact failure time of the ith group. | |||
* <math>S</math> is the number of groups of suspension data points. | |||
* <math>N'_i</math> is the number of suspensions in the ith group of suspension data points. | |||
* <math>T^{'}_{i}</math> is the running time of the ith suspension data group. | |||
* <math>FI</math> is the number of interval data groups. | |||
* is the number of intervals in the ith group of data intervals. | |||
* is the beginning of the ith interval. | |||
* is the ending of the ith interval. | |||
The solution (parameter estimates) will be found by solving for , , so that = 0, = 0 and = 0: | The solution (parameter estimates) will be found by solving for , , so that = 0, = 0 and = 0: | ||
[[Image:chapter8_202.gif|center]] | [[Image:chapter8_202.gif|center]] | ||
Revision as of 18:02, 16 January 2012
IPL-Lognormal
The pdf for the Inverse Power Law relationship and the lognormal distribution is given next.
The pdf of the lognormal distribution is given by:
- [math]\displaystyle{ f(T)=\frac{}{} e^{-\frac{1}{2}(\frac{T'-\overline{T'}}{\sigma_{T'}}^2} }[/math](6)
where:
- [math]\displaystyle{ T'=ln(T) }[/math] = ln(T).
- [math]\displaystyle{ T }[/math] = times-to-failure.
- [math]\displaystyle{ \overline{T}' }[/math] = mean of the natural logarithms of the times-to-failure.
- [math]\displaystyle{ \sigma_{T'} }[/math] = standard deviation of the natural logarithms of the times-to-failure.
The median of the lognormal distribution is given by:
- [math]\displaystyle{ \breve{T}=e^{\overline{T}'} }[/math](7)
The IPL-lognormal model pdf can be obtained first by setting = L(V) in Eqn. ( 30). Therefore:
- [math]\displaystyle{ \breve{T}=L(V)=\frac{1}{K*V^n} }[/math]
or:
- [math]\displaystyle{ e^{\overline{T'}}=\frac{1}{K*V^n} }[/math]
Thus:
- [math]\displaystyle{ \overline{T}'=-ln(K)-n ln(V) }[/math](8)
Substituting Eqn. (8) into Eqn. (6) yields the IPL- lognormal model pdf or:
IPL-Lognormal Statistical Properties Summary
The Mean
The mean life of the IPL-lognormal model (mean of the times-to-failure), , is given by:
(9)
The mean of the natural logarithms of the times-to-failure, , in terms of and is given by:
The Standard Deviation
The standard deviation of the IPL-lognormal model (standard deviation of the times-to-failure), , is given by:
(10)
The standard deviation of the natural logarithms of the times-to-failure, , in terms of and is given by:
The Mode
The mode of the IPL-lognormal model is given by:
IPL-Lognormal Reliability
The reliability for a mission of time T, starting at age 0, for the IPL-lognormal model is determined by:
or:
Reliable Life
The reliable life, or the mission duration for a desired reliability goal, tR is estimated by first solving the reliability equation with respect to time, as follows:
where:
and:
Since = ln(T) the reliable life, tR, is given by:
Lognormal Failure Rate
The lognormal failure rate is given by:
Parameter Estimation
Maximum Likelihood Estimation Method
The complete IPL-lognormal log-likelihood function is:
where:
and:
- Fe is the number of groups of exact times-to-failure data points.
- Ni is the number of times-to-failure data points in the ith time-to-failure data group.
- [math]\displaystyle{ s_{T'} }[/math] is the standard deviation of the natural logarithm of the times-to-failure (unknown, the first of three parameters to be estimated).
- [math]\displaystyle{ K }[/math] is the IPL parameter (unknown, the second of three parameters to be estimated).
- [math]\displaystyle{ n }[/math] is the second IPL parameter (unknown, the third of three parameters to be estimated).
- [math]\displaystyle{ Vi }[/math] is the stress level of the ith group.
- [math]\displaystyle{ Ti }[/math] is the exact failure time of the ith group.
- [math]\displaystyle{ S }[/math] is the number of groups of suspension data points.
- [math]\displaystyle{ N'_i }[/math] is the number of suspensions in the ith group of suspension data points.
- [math]\displaystyle{ T^{'}_{i} }[/math] is the running time of the ith suspension data group.
- [math]\displaystyle{ FI }[/math] is the number of interval data groups.
- is the number of intervals in the ith group of data intervals.
- is the beginning of the ith interval.
- is the ending of the ith interval.
The solution (parameter estimates) will be found by solving for , , so that = 0, = 0 and = 0:
and: