Template:Aaw rf: Difference between revisions
Jump to navigation
Jump to search
(Created page with '====Arrhenius-Weibull Reliability Function==== <br> The Arrhenius-Weibull reliability function is given by: <br> ::<math>R(T,V)={{e}^{-{{\left( \tfrac{T}{C\cdot {{e}^{\tfrac{B}{…') |
|||
| Line 13: | Line 13: | ||
<br> | <br> | ||
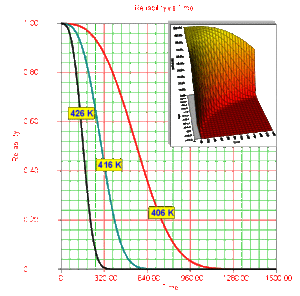
The behavior of the reliability function of the Weibull distribution for different values of <math>\beta </math> was illustrated in | The behavior of the reliability function of the Weibull distribution for different values of <math>\beta </math> was illustrated [[Distributions used in Accelerated Testing#The Weibull Distribution|here]]. In the case of the Arrhenius-Weibull model, however, the reliability is a function of stress also. A 3D plot such as the ones shown in the next figure is now needed to illustrate the effects of both the stress and <math>\beta .</math> | ||
<br> | <br> | ||
Revision as of 23:30, 13 February 2012
Arrhenius-Weibull Reliability Function
The Arrhenius-Weibull reliability function is given by:
- [math]\displaystyle{ R(T,V)={{e}^{-{{\left( \tfrac{T}{C\cdot {{e}^{\tfrac{B}{V}}}} \right)}^{\beta }}}} }[/math]
If the parameter [math]\displaystyle{ B }[/math] is positive, then the reliability increases as stress decreases.
The behavior of the reliability function of the Weibull distribution for different values of [math]\displaystyle{ \beta }[/math] was illustrated here. In the case of the Arrhenius-Weibull model, however, the reliability is a function of stress also. A 3D plot such as the ones shown in the next figure is now needed to illustrate the effects of both the stress and [math]\displaystyle{ \beta . }[/math]
- [math]\displaystyle{ }[/math]