Template:Characteristics of the normal distribution: Difference between revisions
(Created page with '==Characteristics of the Normal Distribution== Some of the specific characteristics of the normal distribution are the following: :• The normal <math>pdf</math> has a mean, …') |
|||
| Line 29: | Line 29: | ||
:• The normal <math>pdf</math> starts at <math>T=-\infty </math> with an <math>f(T)=0</math> . As <math>T</math> increases, <math>f(T)</math> also increases, goes through its point of inflection and reaches its maximum value at <math>T=\bar{T}</math> . Thereafter, <math>f(T)</math> decreases, goes through its point of inflection, and assumes a value of <math>f(T)=0</math> at <math>T=+\infty </math> . | :• The normal <math>pdf</math> starts at <math>T=-\infty </math> with an <math>f(T)=0</math> . As <math>T</math> increases, <math>f(T)</math> also increases, goes through its point of inflection and reaches its maximum value at <math>T=\bar{T}</math> . Thereafter, <math>f(T)</math> decreases, goes through its point of inflection, and assumes a value of <math>f(T)=0</math> at <math>T=+\infty </math> . | ||
'''Weibull++ Notes on Negative Time Values''' | |||
One of the disadvantages of using the normal distribution for reliability calculations is the fact that the normal distribution starts at negative infinity. This can result in negative values for some of the results. Negative values for time are not accepted in most of the components of Weibull++, nor are they implemented. Certain components of the application reserve negative values for suspensions, or will not return negative results. For example, the Quick Calculation Pad will return a null value (zero) if the result is negative. Only the Free-Form (Probit) data sheet can accept negative values for the random variable (x-axis values). | One of the disadvantages of using the normal distribution for reliability calculations is the fact that the normal distribution starts at negative infinity. This can result in negative values for some of the results. Negative values for time are not accepted in most of the components of Weibull++, nor are they implemented. Certain components of the application reserve negative values for suspensions, or will not return negative results. For example, the Quick Calculation Pad will return a null value (zero) if the result is negative. Only the Free-Form (Probit) data sheet can accept negative values for the random variable (x-axis values). | ||
Revision as of 17:43, 10 February 2012
Characteristics of the Normal Distribution
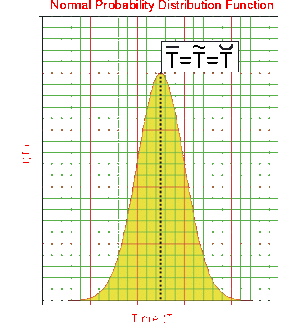
Some of the specific characteristics of the normal distribution are the following:
- • The normal [math]\displaystyle{ pdf }[/math] has a mean, [math]\displaystyle{ \bar{T} }[/math] ,
- which is equal to the median, [math]\displaystyle{ \breve{T} }[/math] ,
- and also equal to the mode, [math]\displaystyle{ \tilde{T} }[/math] ,
- or [math]\displaystyle{ \bar{T}=\breve{T}=\tilde{T} }[/math] .
- and also equal to the mode, [math]\displaystyle{ \tilde{T} }[/math] ,
- which is equal to the median, [math]\displaystyle{ \breve{T} }[/math] ,
This is because the normal distribution is symmetrical about its mean.
- •The mean, [math]\displaystyle{ \mu }[/math] , or the mean life or the [math]\displaystyle{ MTTF }[/math] , is also the location parameter of the normal [math]\displaystyle{ pdf }[/math] , as it locates the [math]\displaystyle{ pdf }[/math] along the abscissa. It can assume values of [math]\displaystyle{ -\infty \lt \bar{T}\lt \infty }[/math] .
- •The normal [math]\displaystyle{ pdf }[/math] has no shape parameter. This means that the normal [math]\displaystyle{ pdf }[/math] has only one shape, the bell shape, and this shape does not change.
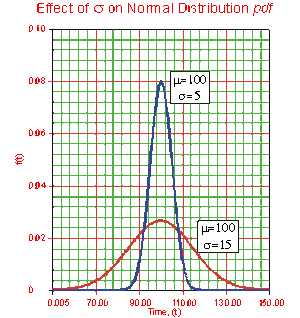
- • The standard deviation, [math]\displaystyle{ {{\sigma }_{T}} }[/math] , is the scale parameter of the normal [math]\displaystyle{ pdf }[/math] .
- • As [math]\displaystyle{ {{\sigma }_{T}} }[/math] decreases, the [math]\displaystyle{ pdf }[/math] gets pushed toward the mean, or it becomes narrower and taller.
- • As [math]\displaystyle{ {{\sigma }_{T}} }[/math] increases, the [math]\displaystyle{ pdf }[/math] spreads out away from the mean, or it becomes broader and shallower.
- • The standard deviation can assume values of [math]\displaystyle{ 0\lt {{\sigma }_{T}}\lt \infty }[/math] .
- • The greater the variability, the larger the value of [math]\displaystyle{ {{\sigma }_{T}} }[/math] , and vice versa.
- • The standard deviation is also the distance between the mean and the point of inflection of the [math]\displaystyle{ pdf }[/math] , on each side of the mean. The point of inflection is that point of the [math]\displaystyle{ pdf }[/math] where the slope changes its value from a decreasing to an increasing one, or where the second derivative of the [math]\displaystyle{ pdf }[/math] has a value of zero.
- • The normal [math]\displaystyle{ pdf }[/math] starts at [math]\displaystyle{ T=-\infty }[/math] with an [math]\displaystyle{ f(T)=0 }[/math] . As [math]\displaystyle{ T }[/math] increases, [math]\displaystyle{ f(T) }[/math] also increases, goes through its point of inflection and reaches its maximum value at [math]\displaystyle{ T=\bar{T} }[/math] . Thereafter, [math]\displaystyle{ f(T) }[/math] decreases, goes through its point of inflection, and assumes a value of [math]\displaystyle{ f(T)=0 }[/math] at [math]\displaystyle{ T=+\infty }[/math] .
Weibull++ Notes on Negative Time Values
One of the disadvantages of using the normal distribution for reliability calculations is the fact that the normal distribution starts at negative infinity. This can result in negative values for some of the results. Negative values for time are not accepted in most of the components of Weibull++, nor are they implemented. Certain components of the application reserve negative values for suspensions, or will not return negative results. For example, the Quick Calculation Pad will return a null value (zero) if the result is negative. Only the Free-Form (Probit) data sheet can accept negative values for the random variable (x-axis values).