Likelihood Ratio Test Example: Difference between revisions
Kate Racaza (talk | contribs) (pulled content from Additional_Tools page) |
Lisa Hacker (talk | contribs) No edit summary |
||
| (4 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
<noinclude>{{Banner ALTA Examples}} | <noinclude>{{Banner ALTA Examples}} | ||
''This example appears in the [ | ''This example appears in the [https://help.reliasoft.com/reference/accelerated_life_testing_data_analysis Accelerated life testing reference].'' | ||
</noinclude> | </noinclude> | ||
| Line 27: | Line 27: | ||
The data set was analyzed using an Arrhenius-Weibull model. The analysis yields: | The data set was analyzed using an Arrhenius-Weibull model. The analysis yields: | ||
::<math>\widehat{\beta }=\ 2.965820\,\!</math> | |||
::<math>\widehat{ | ::<math>\widehat{B}=\ 10,679.567542\,\!</math> | ||
::<math>\widehat{ | ::<math>\widehat{C}=\ 2.396615\cdot {{10}^{-9}}\,\!</math> | ||
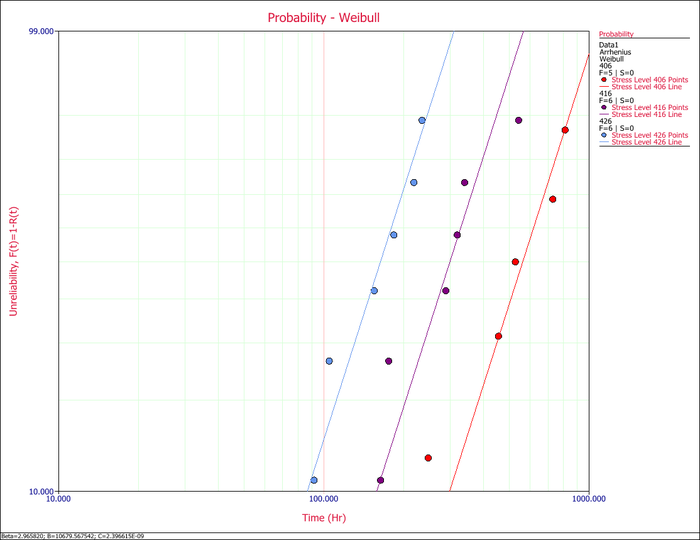
The assumption of a common <math>\beta \,\!</math> across the different stress levels can be visually assessed by using a probability plot. As you can see in the following plot, the plotted data from the different stress levels seem to be fairly parallel. | |||
[[Image:3linedplot.png|center|700px|Probability plot of the three test stress levels.]] | |||
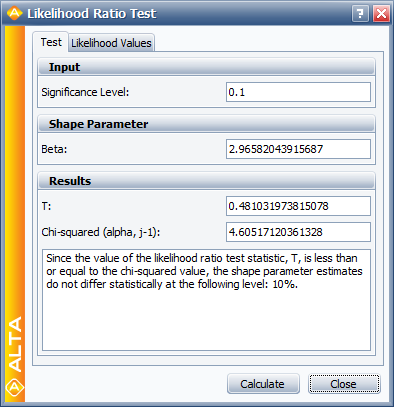
A better assessment can be made with the LR test, which can be performed using the Likelihood Ratio Test tool in ALTA. For example, in the following figure, the <math>\beta s\,\!</math> are compared for equality at the 10% level. | |||
A better assessment can be made with the LR test, which can be performed using the Likelihood Ratio Test tool in ALTA. For example, in the following figure, the | |||
[[Image:lkt.png|center|400px|]] | [[Image:lkt.png|center|400px|]] | ||
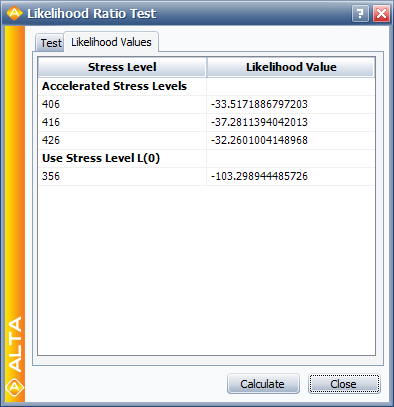
The LR test statistic, <math>T\,\!</math>, is calculated to be 0.481. Therefore, <math>T=0.481\le 4.605={{\chi }^{2}}(0.9;2),\,\!</math> the <math>{\beta }'\,\!</math> s do not differ significantly at the 10% level. The individual likelihood values for each of the test stresses are shown next. | |||
The LR test statistic, | |||
[[Image:lktr.png|center|400px|]] | [[Image:lktr.png|center|400px|]] | ||
Latest revision as of 19:04, 18 September 2023
New format available! This reference is now available in a new format that offers faster page load, improved display for calculations and images and more targeted search.
As of January 2024, this Reliawiki page will not continue to be updated. Please update all links and bookmarks to the latest references at ALTA examples and ALTA reference examples.
This example appears in the Accelerated life testing reference.
Consider the following times-to-failure data at three different stress levels.
| Stress | 406 K | 416 K | 426 K |
|---|---|---|---|
| Time Failed (hrs) | 248 | 164 | 92 |
| 456 | 176 | 105 | |
| 528 | 289 | 155 | |
| 731 | 319 | 184 | |
| 813 | 340 | 219 | |
| 543 | 235 |
The data set was analyzed using an Arrhenius-Weibull model. The analysis yields:
- [math]\displaystyle{ \widehat{\beta }=\ 2.965820\,\! }[/math]
- [math]\displaystyle{ \widehat{B}=\ 10,679.567542\,\! }[/math]
- [math]\displaystyle{ \widehat{C}=\ 2.396615\cdot {{10}^{-9}}\,\! }[/math]
The assumption of a common [math]\displaystyle{ \beta \,\! }[/math] across the different stress levels can be visually assessed by using a probability plot. As you can see in the following plot, the plotted data from the different stress levels seem to be fairly parallel.
A better assessment can be made with the LR test, which can be performed using the Likelihood Ratio Test tool in ALTA. For example, in the following figure, the [math]\displaystyle{ \beta s\,\! }[/math] are compared for equality at the 10% level.
The LR test statistic, [math]\displaystyle{ T\,\! }[/math], is calculated to be 0.481. Therefore, [math]\displaystyle{ T=0.481\le 4.605={{\chi }^{2}}(0.9;2),\,\! }[/math] the [math]\displaystyle{ {\beta }'\,\! }[/math] s do not differ significantly at the 10% level. The individual likelihood values for each of the test stresses are shown next.