Difference Detection Matrix Example: Difference between revisions

From ReliaWiki
Jump to navigation Jump to search
(Undo revision 22945 by Lisa Hacker (Talk))
No edit summary
 
(19 intermediate revisions by 5 users not shown)
Line 1: Line 1:
'''Test Design Using Life Difference Detection Matrix'''  
<noinclude>{{Banner Weibull Examples}}
''This example appears in the [https://help.reliasoft.com/reference/life_data_analysis Life data analysis reference]''.


Assume there are two design options for a new product. Engineers need to design a test to compare the reliability performance of these two options. The reliability for both designs is assumed to follow a Weibull distribution. For Design 1, its shape parameter <span class="texhtml">β</span> = 3; for Design 2, its <span class="texhtml">β</span> = 2. Their B10 lives may range from 500 to 3,000 hours. We want to use the Difference Detection Matrix to choose the suitable sample size and test duration.  
</noinclude>
In this example, you will use the Difference Detection Matrix to choose the suitable sample size and duration for a reliability test. Assume that there are two design options for a new product. The engineers need to design a test that compares the reliability performance of these two options. The reliability for both designs is assumed to follow a Weibull distribution. For Design 1, its shape parameter <math>\beta = 3\,\!</math>; for Design 2, its <math>\beta= 2\,\!</math>. Their B10 lives may range from 500 to 3,000 hours.  


<br> '''Solution'''  
'''Solution'''  


Initially, the sample size for each design is set to 20. Two test durations of 3,000 and 5,000 hours also were chosen. Based on these settings and the assumptions on the failure time distributions, the following Difference Detection Matrix is obtained.  
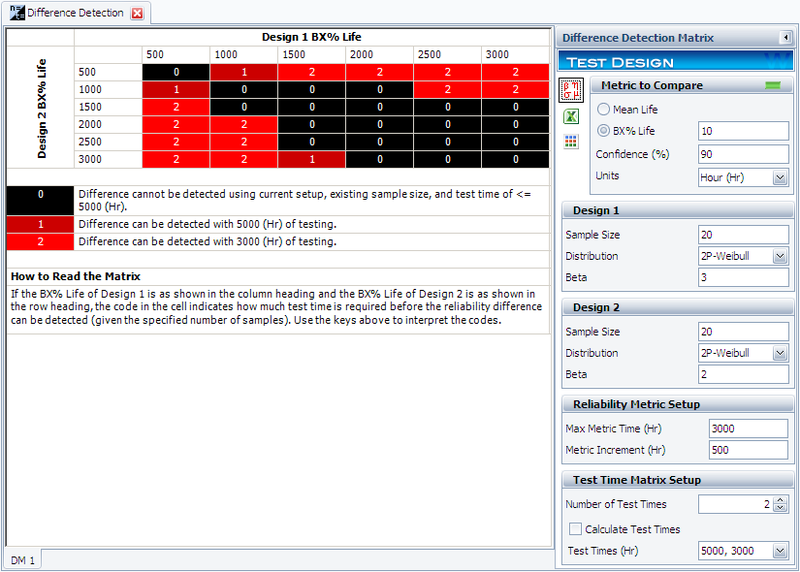
For the initial setup, set the sample size for each design to '''20''', and use two test durations of '''3,000''' and '''5,000''' hours. The following picture shows the complete control panel setup and the results of the analysis.  


[[Image:Detection Matrix Example Result.png|thumb|center|400px]]  
[[Image:Detection Matrix Example Result.png|center|800px]]  


In the figure, the range of the assumed B10 life for each design is from 500 to 3,000 hours. The pair-wised comparison results are displayed in each cell. 0 means the difference cannot be detected through the test; 1 means the difference can be detected if the test duration is 5,000 hours; 2 means the difference can be detected if the test duration is 3,000 hours. For example, the number is 2 for for cell (1000, 2000). This means that if the B10 life for Design 1 is 1,000 hours and the B10 life for Design 2 is 2,000 hours, the difference can be detected if the test duration is at least 5,000 hours. By testing 20 samples each for 3,000 hours, the difference of their B10 lives probably can be detected. This is because, at a confidence level of 90%, the estimated confidence intervals on the B10 life do not overlap. The estimated intervals can be seen in the figure below.  
The columns in the matrix show the range of the assumed B10 life for design 1, while the rows show the range for design 2. A value of 0 means the difference cannot be detected through the test, 1 means the difference can be detected if the test duration is 5,000 hours, and 2 means the difference can be detected if the test duration is 3,000 hours. For example, the number is 2 for cell (1000, 2000). This means that if the B10 life for Design 1 is 1,000 hours and the B10 life for Design 2 is 2,000 hours, the difference can be detected if the test duration is at least 5,000 hours.  


[[Image:Detection Matrix Example B10 Intervals.png|thumb|center|400px]]
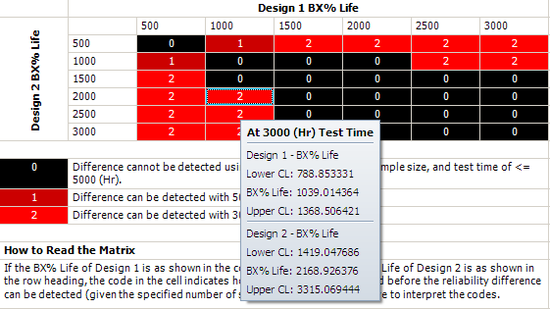
Click inside the cell to show the estimated confidence intervals, as shown next. By testing 20 samples each for 3,000 hours, the difference of their B10 lives probably can be detected. This is because, at a confidence level of 90%, the estimated confidence intervals on the B10 life do not overlap.  
[[Image:Detection Matrix Example B10 Intervals_new.png|thumb|center|400px]]


We will use Design 1 to illustrate how the interval is calculated. For cell (1000, 2000), Design 1's B10 life is 1,000 and the assumed <span class="texhtml">β</span> is 3. We can calculate the <span class="texhtml">η</span> for the Weibull distribution using the '''Quick Parameter Estimator''' tool to calculate eta, as shown next.  
[[Image:Detection Matrix Example B10 Intervals_new.png|center|550px]]


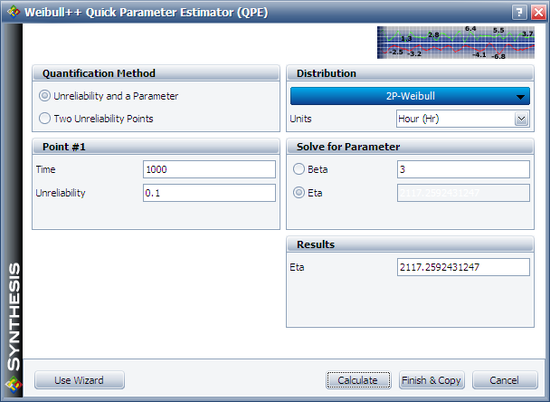
[[Image:Detection Matrix Example Parameter Estimator.png|thumb|center|400px]]
We will use Design 1 to illustrate how the interval is calculated. For cell (1000, 2000), Design 1's B10 life is 1,000 and the assumed <math>\beta\,\!</math> is 3. We can calculate the <math>\eta\,\!</math> for the Weibull distribution using the '''Quick Parameter Estimator''' tool, as shown next.  


The estimated <span class="texhtml">η</span> is 2117.2592 hours. Then we can use these distribution parameters and the sample size of 20 to get the expected failure times using the '''Expected Failure Times Plot''' as shown below.  
[[Image:Detection Matrix Example Parameter Estimator.png|center|550px]]


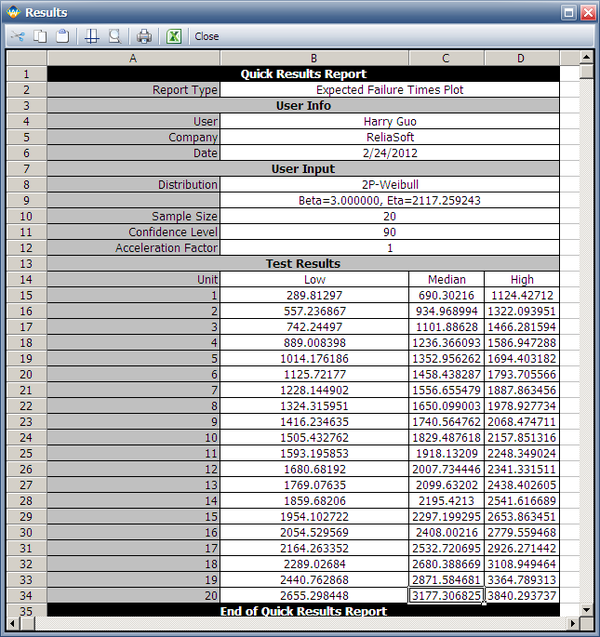
[[Image:Detection Matrix Example Expected Failure Tiime.png|thumb|center|400px]]  
The estimated <math>\eta\,\!</math> is 2117.2592 hours. We can then use these distribution parameters and the sample size of 20 to get the expected failure times by using Weibull's [[Reliability_Test_Design#Expected_Failure_Times_Plots|Expected Failure Times Plot]]. The following report shows the result from that utility.


The median failure times are used to estimate the failure distribution. Please notice that since the test duration is set to 3,000 hours, any failures that occur after 3,000 are treated as suspensions. In this case, the last failure is a suspension with suspension time of 3,000. Now we can enter this data set into a standard Weibull++ folio as given in the next figure.  
[[Image:Detection Matrix Example Expected Failure Tiime.png|center|600px]]


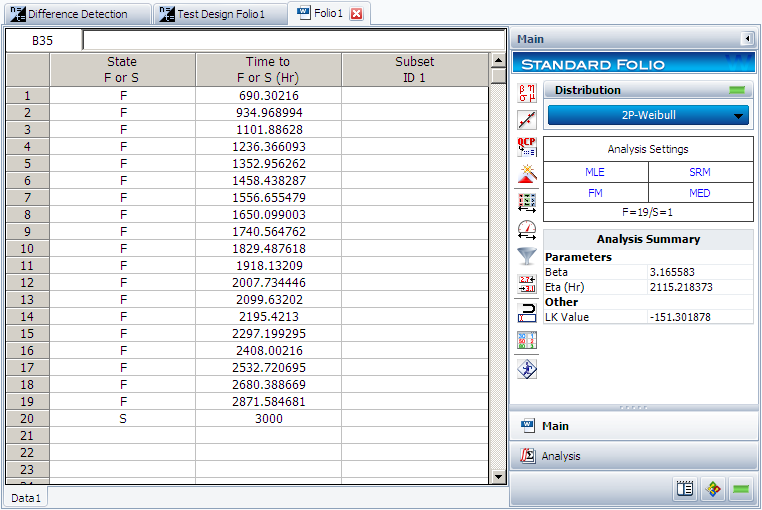
[[Image:Detection Matrix Example Std Folio Data.png.png|thumb|center|400px]]
The median failure times are used to estimate the failure distribution. Note that since the test duration is set to 3,000 hours, any failures that occur after 3,000 are treated as suspensions. In this case, the last failure is a suspension with a suspension time of 3,000 hours. We can enter the median failure times data set into a standard Weibull++ folio as given in the next figure.  


Please make sure the '''MLE''' and '''FM''' options are selected. Using the estimated results, we can calculate the B10 life and its interval in the '''QCP''', as shown below.  
[[Image:Detection Matrix Example Std Folio Data.png.png|center|800px]]


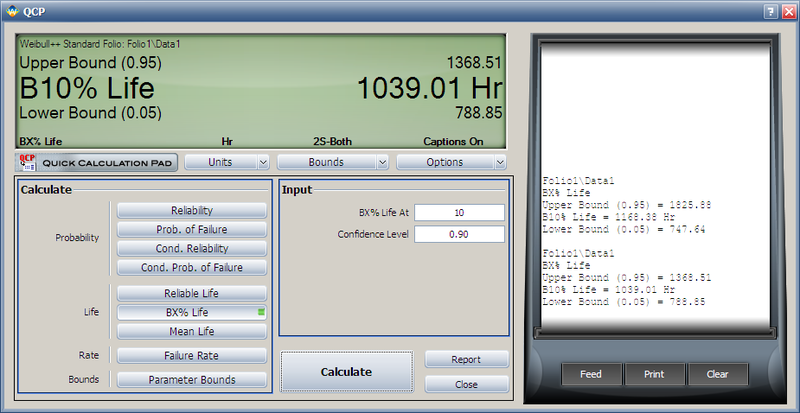
[[Image:Detection Matrix Example B10 QCP.png.png|thumb|center|400px]]
After analyzing the data set with the '''MLE''' and '''FM''' analysis options, we can now calculate the B10 life and its interval in the '''QCP''', as shown next.  


From the above result, we can see the estimated B10 life and its confidence intervals are the same as the results displayed in the Difference Detection Matrix.  
[[Image:Detection Matrix Example B10 QCP.png.png|center|800px]]
 
From this result, we can see that the estimated B10 life and its confidence intervals are the same as the results displayed in the Difference Detection Matrix.  


The above procedure can be repeated to get the results for the other cells and for Design 2. Therefore, by adjusting the sample size and test duration, a suitable test time can be identified for detecting a certain amount of difference between two designs/populations.
The above procedure can be repeated to get the results for the other cells and for Design 2. Therefore, by adjusting the sample size and test duration, a suitable test time can be identified for detecting a certain amount of difference between two designs/populations.

Latest revision as of 18:56, 18 September 2023

Weibull Examples Banner.png


New format available! This reference is now available in a new format that offers faster page load, improved display for calculations and images and more targeted search.

As of January 2024, this Reliawiki page will not continue to be updated. Please update all links and bookmarks to the latest references at Weibull examples and Weibull reference examples.



This example appears in the Life data analysis reference.


In this example, you will use the Difference Detection Matrix to choose the suitable sample size and duration for a reliability test. Assume that there are two design options for a new product. The engineers need to design a test that compares the reliability performance of these two options. The reliability for both designs is assumed to follow a Weibull distribution. For Design 1, its shape parameter [math]\displaystyle{ \beta = 3\,\! }[/math]; for Design 2, its [math]\displaystyle{ \beta= 2\,\! }[/math]. Their B10 lives may range from 500 to 3,000 hours.

Solution

For the initial setup, set the sample size for each design to 20, and use two test durations of 3,000 and 5,000 hours. The following picture shows the complete control panel setup and the results of the analysis.

Detection Matrix Example Result.png

The columns in the matrix show the range of the assumed B10 life for design 1, while the rows show the range for design 2. A value of 0 means the difference cannot be detected through the test, 1 means the difference can be detected if the test duration is 5,000 hours, and 2 means the difference can be detected if the test duration is 3,000 hours. For example, the number is 2 for cell (1000, 2000). This means that if the B10 life for Design 1 is 1,000 hours and the B10 life for Design 2 is 2,000 hours, the difference can be detected if the test duration is at least 5,000 hours.

Click inside the cell to show the estimated confidence intervals, as shown next. By testing 20 samples each for 3,000 hours, the difference of their B10 lives probably can be detected. This is because, at a confidence level of 90%, the estimated confidence intervals on the B10 life do not overlap.

Detection Matrix Example B10 Intervals new.png

We will use Design 1 to illustrate how the interval is calculated. For cell (1000, 2000), Design 1's B10 life is 1,000 and the assumed [math]\displaystyle{ \beta\,\! }[/math] is 3. We can calculate the [math]\displaystyle{ \eta\,\! }[/math] for the Weibull distribution using the Quick Parameter Estimator tool, as shown next.

Detection Matrix Example Parameter Estimator.png

The estimated [math]\displaystyle{ \eta\,\! }[/math] is 2117.2592 hours. We can then use these distribution parameters and the sample size of 20 to get the expected failure times by using Weibull's Expected Failure Times Plot. The following report shows the result from that utility.

Detection Matrix Example Expected Failure Tiime.png

The median failure times are used to estimate the failure distribution. Note that since the test duration is set to 3,000 hours, any failures that occur after 3,000 are treated as suspensions. In this case, the last failure is a suspension with a suspension time of 3,000 hours. We can enter the median failure times data set into a standard Weibull++ folio as given in the next figure.

Detection Matrix Example Std Folio Data.png.png

After analyzing the data set with the MLE and FM analysis options, we can now calculate the B10 life and its interval in the QCP, as shown next.

Detection Matrix Example B10 QCP.png.png

From this result, we can see that the estimated B10 life and its confidence intervals are the same as the results displayed in the Difference Detection Matrix.

The above procedure can be repeated to get the results for the other cells and for Design 2. Therefore, by adjusting the sample size and test duration, a suitable test time can be identified for detecting a certain amount of difference between two designs/populations.

Retrieved from "https://www.reliawiki.com/index.php?title=Difference_Detection_Matrix_Example&oldid=66020"