Lloyd-Lipow Confidence Bounds Example

New format available! This reference is now available in a new format that offers faster page load, improved display for calculations and images and more targeted search.

As of January 2024, this Reliawiki page will not continue to be updated. Please update all links and bookmarks to the latest references at RGA examples and RGA reference examples.

---

This example appears in the Reliability growth reference.

Consider the success/failure data given in the following table. Solve for the Lloyd-Lipow parameters using least squares analysis, and plot the Lloyd-Lipow reliability with 2-sided confidence bounds at the 90% confidence level.

Success/Failure Data for a Variable Number of Tests Performed in Each Test Stage

Test Stage Number([math]\displaystyle{ k\,\! }[/math])	Result	Number of Tests([math]\displaystyle{ n_k\,\! }[/math]>	Successful Tests([math]\displaystyle{ S_k=R_i\,\! }[/math])
1	F	1	0
2	F	1	0
3	F	1	0
4	S	1	0.2500
5	F	1	0.2000
6	F	1	0.1667
7	S	1	0.2857
8	S	1	0.3750
9	S	1	0.4444
10	S	1	0.5000
11	S	1	0.5455
12	S	1	0.5833
13	S	1	0.6154
14	S	1	0.6429
15	S	1	0.6667
16	S	1	0.6875
17	F	1	0.6471
18	S	1	0.6667
19	F	1	0.6316
20	S	1	0.6500
21	S	1	0.6667
22	S	1	0.6818

Solution

Note that the data set contains three consecutive failures at the beginning of the test. These failures will be ignored throughout the analysis because it is considered that the test starts when the reliability is not equal to zero or one. The number of data points is now reduced to 19. Also, note that the only time that the first three first failures are considered is to calculate the observed reliability in the test. For example, given this data set, the observed reliability at stage 4 is [math]\displaystyle{ 1/4=0.25\,\! }[/math]. This is considered to be the reliability at stage 1.

From the table, the least squares estimates can be calculated as follows:

[math]\displaystyle{ \begin{align} \underset{k=1}{\overset{N}{\mathop \sum }}\,\frac{1}{k}= & \underset{k=1}{\overset{19}{\mathop \sum }}\,\frac{1}{k}=3.54774 \\ \underset{k=1}{\overset{N}{\mathop \sum }}\,\frac{1}{{{k}^{2}}}= & \underset{k=1}{\overset{19}{\mathop \sum }}\,\frac{1}{{{k}^{2}}}=1.5936 \\ \underset{k=1}{\overset{N}{\mathop \sum }}\,\frac{{{S}_{k}}}{{{n}_{k}}}= & \underset{k=1}{\overset{19}{\mathop \sum }}\,\frac{{{S}_{k}}}{{{n}_{k}}}=9.907 \end{align}\,\! }[/math]

and:

[math]\displaystyle{ \underset{k=1}{\overset{N}{\mathop \sum }}\,\frac{{{S}_{k}}}{k\cdot {{n}_{k}}}=\underset{k=1}{\overset{19}{\mathop \sum }}\,\frac{{{S}_{k}}}{k\cdot {{n}_{k}}}=1.3002\,\! }[/math]

Using these estimates to obtain [math]\displaystyle{ \hat{R}_{\infty}\,\! }[/math] and [math]\displaystyle{ \hat{\alpha}\,\! }[/math] yields:

[math]\displaystyle{ \begin{align} {{{\hat{R}}}_{\infty }} = & \frac{(1.5936)(9.907)-(3.5477)(1.3002)}{(19)(1.5936)-{{(3.5477)}^{2}}} \\ = & 0.6316 \end{align}\,\! }[/math]

and:

[math]\displaystyle{ \begin{align} \hat{\alpha } = & \frac{(3.5477)(9.907)-(19)(1.3002)}{(19)(1.5936)-{{(3.5477)}^{2}}} \\ = & 0.5902 \end{align}\,\! }[/math]

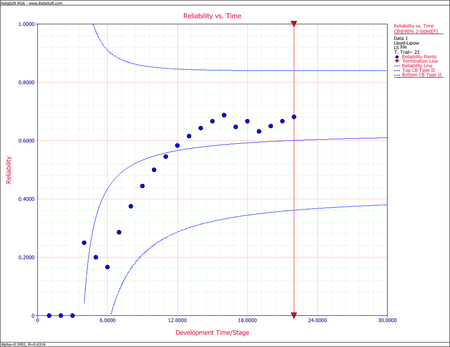
Therefore, the Lloyd-Lipow reliability growth model is as follows, where [math]\displaystyle{ k\,\! }[/math] is the number of the test stage.

[math]\displaystyle{ {{R}_{k}}=0.6316-\frac{0.5902}{k}\,\! }[/math]

Using the data from the table:

[math]\displaystyle{ \begin{align} \frac{{{\partial }^{2}}\Lambda }{\partial R_{\infty }^{2}} = & -176.847-40.500=-217.347 \\ \frac{{{\partial }^{2}}\Lambda }{\partial {{\alpha }^{2}}} = & -146.763-2.1274=-148.891 \\ \frac{{{\partial }^{2}}\Lambda }{\partial {{R}_{\infty }}\partial \alpha } = & 149.909-6.5660=143.343 \end{align}\,\! }[/math]

The variances can be calculated using the Fisher Matrix:

[math]\displaystyle{ \begin{align} {{\left[ \begin{matrix} 217.347 & -143.343 \\ -143.343 & 148.891 \\ \end{matrix} \right]}^{-1}}= & \left[ \begin{matrix} Var({{\widehat{R}}_{\infty }}) & Cov({{\widehat{R}}_{\infty }},\widehat{\alpha }) \\ Cov({{\widehat{R}}_{\infty }},\widehat{\alpha }) & Var(\widehat{\alpha }) \\ \end{matrix} \right] \\ = & \left[ \begin{matrix} 0.0126033 & 0.0121335 \\ 0.0121335 & 0.0183977 \\ \end{matrix} \right] \end{align}\,\! }[/math]

The variance of [math]\displaystyle{ {{R}_{k}}\,\! }[/math] is therefore:

[math]\displaystyle{ Var({{\widehat{R}}_{k}})=0.0126031+\frac{1}{{{k}^{2}}}\cdot 0.0183977-\frac{2}{k}\cdot 0.0121335\,\! }[/math]

The confidence bounds on reliability can now can be calculated. The associated confidence bounds on reliability at the 90% confidence level are plotted in the following figure, with the predicted reliability, [math]\displaystyle{ {{R}_{k}}\,\! }[/math].