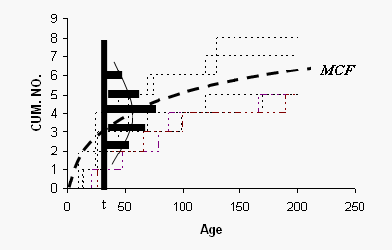Template:Mean cumulative function for recurrence data: Difference between revisions
Kate Racaza (talk | contribs) No edit summary |
Kate Racaza (talk | contribs) No edit summary |
||
| Line 1: | Line 1: | ||
=== The Mean Cumulative Function (MCF) for | === The Mean Cumulative Function (MCF) for Recurrent Event Data Analysis === | ||
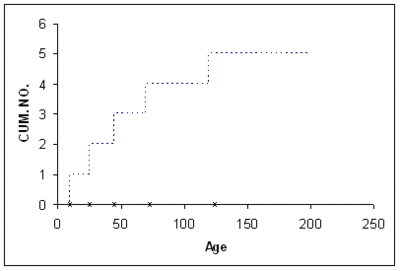
In non-parametric analysis of recurrent | In a non-parametric analysis of recurrent event data, each population unit can be described by a cumulative history function for the cumulative number of recurrences. It is a staircase function that depicts the cumulative number of recurrences of a particular event, such as repairs over time. The figure below depicts a unit's cumulative history function. | ||
[[Image:Lda11.1.gif|thumb|center|400px]] | [[Image:Lda11.1.gif|thumb|center|400px]] | ||
| Line 24: | Line 24: | ||
<span class="texhtml">''N''</span> is the total number of units and <span class="texhtml">''r''<sub>1</sub> = ''N''</span> at the first observed age which could be a recurrence or suspension. | <span class="texhtml">''N''</span> is the total number of units and <span class="texhtml">''r''<sub>1</sub> = ''N''</span> at the first observed age which could be a recurrence or suspension. | ||
'''3rd Step - Calculate the <span class="texhtml">''M | '''3rd Step - Calculate the <span class="texhtml">''M''</span><span class="texhtml"></span>C''''F''''''estimate, <span class="texhtml"></span>:'''For each sample recurrence age <span class="texhtml"></span> calculate the mean cumulative function estimate as follows:'''''M''<sup>* </sup>(''t'')'''''t''<sub></sub>,''''' | ||
::<math>{{M}^{*}}({{t}_{i}})=\frac{1}{{{r}_{i}}}+{{M}^{*}}({{t}_{i-1}})</math> | ::<math>{{M}^{*}}({{t}_{i}})=\frac{1}{{{r}_{i}}}+{{M}^{*}}({{t}_{i-1}})</math> | ||
Revision as of 16:27, 8 March 2012
The Mean Cumulative Function (MCF) for Recurrent Event Data Analysis
In a non-parametric analysis of recurrent event data, each population unit can be described by a cumulative history function for the cumulative number of recurrences. It is a staircase function that depicts the cumulative number of recurrences of a particular event, such as repairs over time. The figure below depicts a unit's cumulative history function.
The non-parametric model for a population of units is described as the population of cumulative history functions (curves). It is the population of all staircase functions of every unit in the population. At age t, the units have a distribution of their cumulative number of events. That is, a fraction of the population has accumulated 0 recurrences, another fraction has accumulated 1 recurrence, another fraction has accumulated 2 recurrences, etc. This distribution differs at different ages t , and has a mean M(t) called the mean cumulative function (MCF). The M(t) is the point-wise average of all population cumulative history functions (see figure below).
For the case of uncensored data, the mean cumulative function [math]\displaystyle{ M{{(t)}_{i}}\ }[/math] values at different recurrence ages ti are estimated by calculating the average of the cumulative number of recurrences of events for each unit in the population at ti . When the histories are censored, the following steps are applied.
1st Step - Order all ages:
Order all recurrence and censoring ages from smallest to largest. If a recurrence age for a unit is the same as its censoring (suspension) age, then the recurrence age goes first. If multiple units have a common recurrence or censoring age, then these units could be put in a certain order or be sorted randomly.
2nd Step - Calculate the number, ri , of units that passed through age ti :
- [math]\displaystyle{ \begin{align} & {{r}_{i}}= & {{r}_{i-1}}\quad \quad \text{if }{{t}_{i}}\text{ is a recurrence age} \\ & {{r}_{i}}= & {{r}_{i-1}}-1\text{ if }{{t}_{i}}\text{ is a censoring age} \end{align} }[/math]
N is the total number of units and r1 = N at the first observed age which could be a recurrence or suspension.
3rd Step - Calculate the MC'F'estimate, :For each sample recurrence age calculate the mean cumulative function estimate as follows:M* (t)t,
- [math]\displaystyle{ {{M}^{*}}({{t}_{i}})=\frac{1}{{{r}_{i}}}+{{M}^{*}}({{t}_{i-1}}) }[/math]
where [math]\displaystyle{ {{M}^{*}}(t)=\tfrac{1}{{{r}_{1}}} }[/math] at the earliest observed recurrence age, t1 .
Example 1: Template:Example: Recurrent Events Data Non-Parameteric MCF Example